MY-ASSIGNMENTEXPERT™可以为您提供ntnu.edu TEP4120 Thermodynamics热力学课程的代写代考和辅导服务!
这是挪威科技大学 热力学课程的代写成功案例。
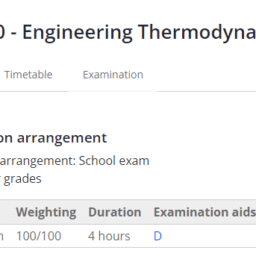
TEP4120课程简介
Course content
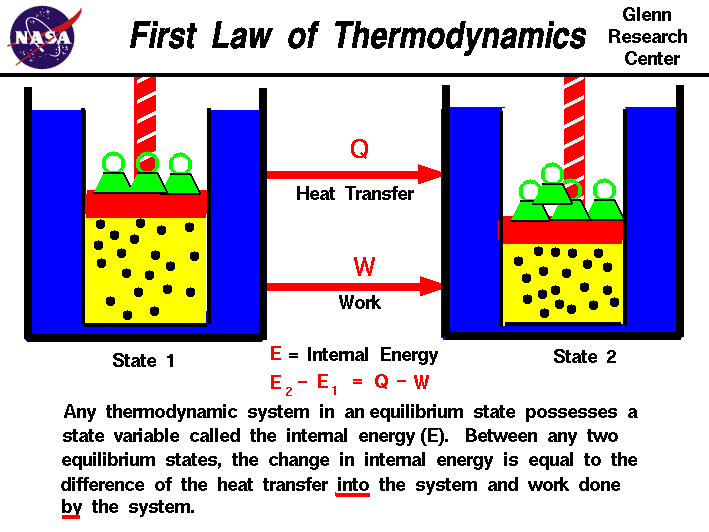
Concepts and definitions; the thermodynamic system, properties, phase equilibrium of pure substances, equations of state for gases, tables of thermodynamic properties, work and heat. First law of thermodynamics; thermodynamic cycles, change of state, internal energy, enthalpy, specific heat; open systems, steady-state and transient processes. Second law of thermodynamics; reversible and irreversible processes, the Carnot cycle, the thermodynamic temperature scale, entropy, the entropy production concept. Thermodynamic power cycles, refrigeration cycles, the Otto cycle and the Diesel cycle, the gas-turbine process. Introduction to exergy analysis.
Prerequisites
LKnowledge: The course provides the student with knowledge about: – Conservation laws for mass and energy (including the 1st law of Thermodynamics). – Forms of energy such as work (power) and heat, internal energy and enthalpy. – Entropy and the 2nd law of thermodynamics. – Reasons for thermodynamic losses in the form of irreversibilities. – The quality of different forms of energy measured as the ability to produce work. – Destruction of energy quality in processes. – Ideal gas model, its assumptions, applications and limitations. – Different cyclic processes such as Carnot, Rankine, Otto, Diesel and Brayton. The course gives the student insight about: – Operation of steam and gas based power stations, internal combustion engines, heat pumps and refrigeration cycles. – The main components of heat & power processes, such as steam and gas turbines, compressors, pumps, fans, heat exchangers and valves. – The ability of fluids to change phase (solid, liquid and gas).
TEP4120 Thermodynamics HELP(EXAM HELP, ONLINE TUTOR)
A corollary which follows from the Carathéodory statement of the second law is,
In the space of thermodynamic equilibrium states, no two reversible adiabatic (hyper) surfaces can ever intersect.
Prove this by contradiction by repeating the argument in the class, i.e. if the opposite holds then the Kelvin-Planck version of the second law is violated.
5 points
In the lecture(s) while proving that the integrating factor for the heat pfaffian is a function of the temperature, $\theta$, we set up a thought experiment where we had a composite system made up of a main system, $A$ and reference system $B$. We deduced from the equations,
$$
\frac{\lambda}{\Lambda}=\frac{\partial \Sigma}{\partial \sigma}, \frac{\lambda^{\prime}}{\Lambda}=\frac{\partial \Sigma}{\partial \sigma^{\prime}}
$$
that the integrating factors for the main system, the reference system and the composite system must be of the forms:
$$
\begin{aligned}
\lambda & =\varphi(\theta) f(\sigma) \
\lambda^{\prime} & =\varphi(\theta) f^{\prime}\left(\sigma^{\prime}\right) \
\Lambda & =\varphi(\theta) g\left(\sigma, \sigma^{\prime}\right)
\end{aligned}
$$
Prove that $g\left(\sigma, \sigma^{\prime}\right)=g\left(\Sigma\left(\sigma, \sigma^{\prime}\right)\right)$. Hint: Show that the Jacobian of the transformation from $\sigma, \sigma^{\prime}$ to $g\left(\sigma, \sigma^{\prime}\right), \Sigma\left(\sigma, \sigma^{\prime}\right)$ vanishes.
5 points
Show that the following Pfaffian form (of three variables $x ; y ; z$ ) does not admit an integrating factor:
$$
d F=x d y+k d z
$$
where $k$ is a constant. (Hint: Refer to the note on exact and inexact differentials posted on the website).
5 points
For an ideal gas with constant heat capacities, show that:
A. The entropy can be given by the expression
$$
S=C_V \ln P+C_P \ln V+\text { const. }
$$
B. The adiabatic compressibility is
$$
\kappa_S \equiv-\frac{1}{V}\left(\frac{\partial V}{\partial P}\right)_S=\frac{1}{\gamma P}
$$
where $\gamma=C_P / C_V$
$$
5+2=7 \text { points }
$$

MY-ASSIGNMENTEXPERT™可以为您提供CATALOG.WINONA.EDU PHYS451 QUANTUM MECHANICS量子力学课程的代写代考和辅导服务