如果你也在 怎样代写复杂网络Complex Network 这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。复杂网络Complex Network在网络理论的背景下,复杂网络是指具有非微观拓扑特征的图(网络)–这些特征在简单的网络(如格子或随机图)中不会出现,但在代表真实系统的网络中经常出现。复杂网络的研究是一个年轻而活跃的科学研究领域(自2000年以来),主要受到现实世界网络的经验发现的启发,如计算机网络、生物网络、技术网络、大脑网络、气候网络和社会网络。
复杂网络Complex Network大多数社会、生物和技术网络显示出实质性的非微观拓扑特征,其元素之间的连接模式既不是纯粹的规则也不是纯粹的随机。这些特征包括学位分布的重尾、高聚类系数、顶点之间的同态性或异态性、社区结构和层次结构。在有向网络的情况下,这些特征还包括互惠性、三联体重要性概况和其他特征。相比之下,过去研究的许多网络的数学模型,如格子和随机图,并没有显示这些特征。最复杂的结构可以由具有中等数量相互作用的网络实现。这与中等概率获得最大信息含量(熵)的事实相对应。
复杂网络Complex Network代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。最高质量的复杂网络Complex Network作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此复杂网络Complex Network作业代写的价格不固定。通常在专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
同学们在留学期间,都对各式各样的作业考试很是头疼,如果你无从下手,不如考虑my-assignmentexpert™!
my-assignmentexpert™提供最专业的一站式服务:Essay代写,Dissertation代写,Assignment代写,Paper代写,Proposal代写,Proposal代写,Literature Review代写,Online Course,Exam代考等等。my-assignmentexpert™专注为留学生提供Essay代写服务,拥有各个专业的博硕教师团队帮您代写,免费修改及辅导,保证成果完成的效率和质量。同时有多家检测平台帐号,包括Turnitin高级账户,检测论文不会留痕,写好后检测修改,放心可靠,经得起任何考验!
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
数据科学代写|复杂网络代写Complex Network代考|Evolutionary Stability of Half-Chaotic Networks
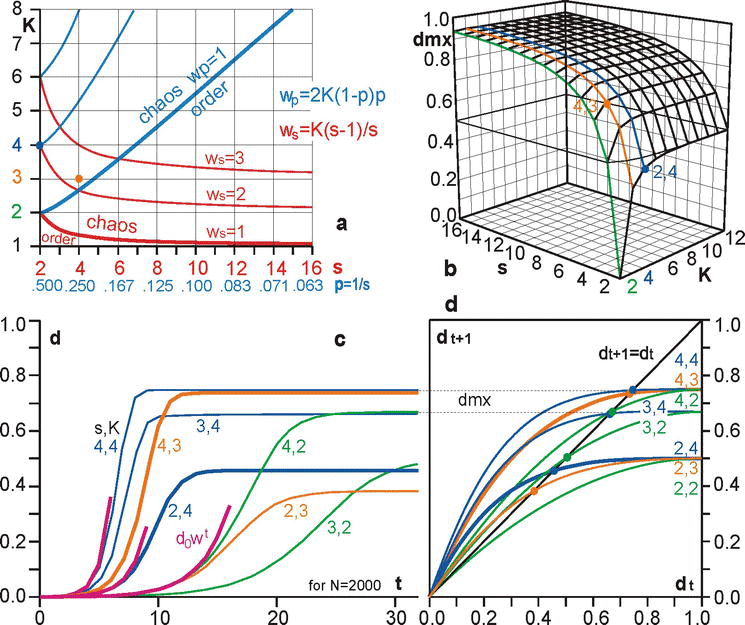
In the first round of earlier experiments PAS networks were investigated, and half-chaotic systems have been explored. In this work, a new disturbance was made from the same initial network state in order to measure the system’s type (ordered, half-chaotic or chaotic). From this finding, questions arise: What conditions must evolutionary changes meet in order to remain in the halfchaotic state? How long can evolution continue within half-chaos? Acceptation (as an evolutionary change) of one small disturbance leading to large damage (i.e. chaotic changes-in the range of the right peak) moves the system into normal chaos. However, when the response to such a disturbance is ordered (in the range of left peak), then the majority of networks created (by that small disturbance) are also PAS $(>99 \%)$. The evolution from PAS to PAS can be infinite; but such a model of evolution is unsatisfactory, because it does not reflect the complexities and dynamic properties of the observed real-world phenomena.
Therefore, in the next experiments (5, 6 and 7 ), evolution was investigated without accepting PAS. In other words, disturbances leading to small damage were accepted as evolutionary changes, only when the new attractor path was $\geq 7$. Note, that PAS has attractor path $=1$. This change was intended to more rapidly move the system away from PAS towards chaos, but the process always stabilised in half-chaos. Limiting the evolution of half-chaotic system to changes causing small damage is enough to remain in half-chaos. This property is called the evolutionary stability of half-chaos.
In experiment 5 , the new evolution was started from PAS, which is an exceptional case for our networks. The whole network was frozen, with each node iced. In other words, their states were not changing over time. Most changes normally accepted in PAS are also PAS. This is the case for over $99 \%$ of accepted cases, across various network types and parameters $s$ and $K$. This type of system evolution can be quite long before something interesting takes place. Therefore, to force the system to move out of PAS, we have introduced the concept of cumulative changes, which is defined as changes that lead to an attractor of length of at least 7 . Those changes are left in the network and contribute to the evolution, whereas changes that lead to attractors of shorter length, are ignored. Using this kind of cumulative change, in the first step of evolution, PAS disappeared, but most of the nodes stayed iced, with only a small unfrozen lakes of activity emerging. This is similar to Kauffman’s description of a liquid area, where he saw life, but it was limited to areas near $s=2$ and $K=2$, whereas in half-chaos it is also observed for $s>2$ and $K>2$.
数据科学代写|复杂网络代写Complex Network代考|Conclusions
Kauffman considered Random Boolean networks (RBNs) and on their basis made his hypothesis limiting connectivity to $K=2$, and number of signal variants to $s=2$. However, those parameters are limiting and do not allow exploration of all possible network state regimes, that has been demonstrated in our own experiments. The process of natural evolution and naturally occurring phenomena exceed these conservative limits. RBN also lay the basis of theories regarding the Gene Regulatory network. Their properties were mainly investigated by mathematicians and compared with the results of experiments on the genome of real living objects. Despite receiving some agreement, doubts remained, as the evaluation of connectivity $K$ in the experimental results did not meet the limitations resulting from the Kauffman hypothesis. GRN was, however, an appealing concept and was picked up by many biologists and is still in use today. Most authors do not go into the basics of the model and its deeper connections with the hypothesis, but simply used the conclusions of the model.
Overall, the simplistic Kauffman model exhibits severe limitations as demonstrated by our current and previous experimental results. In 2003 Banzhaf presented a competitive model of gene regulation much closer to the phenomena occurring in the natural processes of mutation and gene reading. Banzhaf model focuses on and describes quite different aspects of regulation and it does not belong to the same category of models.
Half-chaos introduces real, objective threshold of damage size leading to losing of stability and identity of modelled system, absent in Kauffman’s model. This in turn should revitalise the current model and provide biologists with a larger and more experiential range of conclusions and allow them to re-examine models of GRN and extend them to new ranges of parameters.
The parameters $s$ and $K$ in the range where the random networks are chaotic, are a necessary prerequisite for the explanation of structural tendencies . Structural tendencies is an important phenomenon that corresponds to the regularities of the evolution of ontogenesis.
复杂网络代写
数据科学代写|复杂网络代写Complex Network代考|Evolutionary Stability of Half-Chaotic Networks
在早期的第一轮实验中,研究了PAS网络,并探索了半混沌系统。在这项工作中,为了测量系统的类型(有序、半混沌或混沌),从相同的初始网络状态制造一个新的扰动。根据这一发现,问题出现了:为了保持半混沌状态,进化变化必须满足什么条件?在半混沌状态下进化还能持续多久?接受(作为一种进化变化)一个小的扰动导致大的破坏(即混沌变化-在正确的峰值范围内)使系统进入正常的混沌。然而,当对这种扰动的响应是有序的(在左峰范围内),那么(由那个小扰动)创建的大多数网络也是PAS $(>99 \%)$。从PAS到PAS的演变可以是无限的;但这样的进化模型是不令人满意的,因为它不能反映观察到的现实世界现象的复杂性和动态特性。
因此,在接下来的实验(5、6和7)中,我们将在不接受PAS的情况下研究进化。换句话说,只有当新的吸引子路径为$\geq 7$时,导致小破坏的干扰才被认为是进化变化。注意,PAS有吸引子路径$=1$。这种改变是为了更快地将系统从PAS转向混乱,但这个过程总是在半混乱中稳定下来。将半混沌系统的演化限制在造成小伤害的变化上,就足以保持半混沌状态。这种性质被称为半混沌的演化稳定性。在实验5中,新的进化是从PAS开始的,这是我们网络的例外情况。整个网络被冻结,每个节点都被冻结。换句话说,它们的状态不会随着时间而改变。PAS中通常接受的大多数更改也是PAS。对于超过$99 \%$的可接受案例,跨越各种网络类型和参数$s$和$K$,情况就是如此。在有趣的事情发生之前,这种类型的系统进化可能需要相当长的时间。因此,为了迫使系统移出PAS,我们引入了累积变化的概念,它被定义为导致长度至少为7的吸引子的变化。这些变化留在网络中并促进进化,而导致较短长度吸引子的变化则被忽略。利用这种累积变化,在进化的第一步,PAS消失了,但大多数节点保持结冰,只有一小部分未结冰的活动湖泊出现。这与考夫曼对液体区域的描述类似,他在那里看到了生命,但它仅限于$s=2$和$K=2$附近的区域,而在半混沌中,$s>2$和$K>2$也观察到生命。
数据科学代写|复杂网络代写Complex Network代考|Conclusions
考夫曼考虑了随机布尔网络(rbn),并在其基础上提出了他的假设,将连通性限制在$K=2$,信号变体的数量限制在$s=2$。然而,这些参数是有限的,并且不允许探索所有可能的网络状态制度,这已经在我们自己的实验中得到了证明。自然进化的过程和自然发生的现象超出了这些保守的界限。RBN也为基因调控网络的理论研究奠定了基础。它们的性质主要由数学家研究,并与真实生物基因组的实验结果进行比较。尽管获得了一些共识,但怀疑仍然存在,因为实验结果中对连通性$K$的评估不符合Kauffman假设的限制。然而,GRN是一个吸引人的概念,被许多生物学家采纳,至今仍在使用。大多数作者没有深入研究模型的基础及其与假设的更深层次的联系,而只是简单地使用模型的结论。
总的来说,简单的考夫曼模型显示出严重的局限性,正如我们目前和以前的实验结果所证明的那样。2003年,Banzhaf提出了一种更接近于突变和基因读取自然过程中发生的现象的竞争性基因调控模型。Banzhaf模型关注并描述了监管的不同方面,它不属于同一类别的模型。
半混沌引入了真实的、客观的损伤大小阈值,导致被建模系统失去稳定性和身份,这在Kauffman模型中是不存在的。这反过来应该使当前的模型恢复活力,并为生物学家提供更大、更有经验的结论范围,并允许他们重新检查GRN模型并将其扩展到新的参数范围。
随机网络混沌范围内的参数$s$和$K$是解释结构趋势的必要前提。结构倾向是一种与个体发生演化规律相对应的重要现象。

数据科学代写|复杂网络代写Complex Network代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。