数学代写代考| Simple and Compound Interest 离散数学代写 - 代考代写：100%准时可靠您的作业代写专家

Savers receive interest on placing deposits at the bank for a period of time, whereas lenders pay interest on their loans to the bank. We distinguish between simple and compound interest, where simple interest is always calculated on the original principal, whereas for compound interest, the interest is added to the principal sum, so that interest is also earned on the added interest for the next compounding period. For example, if Euro 1000 is placed on deposit at a bank with an interest rate of $10 \%$ per annum for 2 years, it would earn a total of Euro 200 in simple interest. The interest amount is calculated by
$$
\frac{1000 * 10 * 2}{100}=\text { Euro } 200 \text {. }
$$
The general formula for calculating simple interest on principal $P$, at a rate of interest $I$, and for time $T$ (in years:), is
$$
A=\frac{P \times I \times T}{100} .
$$
The calculation of compound interest is more complicated as may be seen from the following example.
Example (Compound Interest)
Calculate the interest earned and what the new principal will be on Euro 1000 , which is placed on deposit at a bank, with an interest rate of $10 \%$ per annum (compound) for 3 years.
Solution
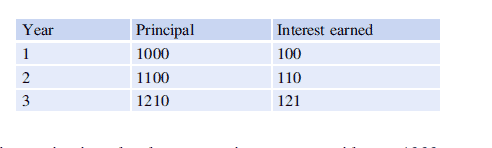
At the end of year 1, Euro 100 of interest is earned, and this is capitalized making the new principal at the start of year 2 Euro 1100 . At the end of year 2, Euro 110 is earned in interest, making the new principal at the start of year 3 Euro $1210 .$ Finally, at the end of year 3, a further Euro 121 is earned in interest, and so the new principal is Euro 1331 and the total interest earned for the 3 years is the sum of the interest earned for each year (i.e. Euro 331). This may be seen from Table $5.1 .$
The new principal each year is given by the geometric sequence with $a=1000$ and $r=10 / 100=0.1$.
$$
1000,1000(1.1), 1000(1.1)^{2}, 1000(1.1)^{3}, \ldots
$$
94
5 Sequences, Series, and Permutations and Combinations Table $5.1$ Calculation of compound interest compound interest
In general, if a principal amount $P$ is invested is expressed as a proportion, i.e. $\left.r={ }^{R} / 100\right)$, the $$ \mathrm{A}=P(1+r)^{T} . $$
For our example above, $A=1000, T=3$ and $r=0.1$ Therefore,
$$
\begin{aligned}
A &=1000(1.1)^{3} \
&=1331(\text { as before })
\end{aligned}
$$
There are variants of the compound interest formula to cover situations where there are $m$-compounding periods per year, and so the reader may consult the available texts. We discuss basic financial mathematics in more detail in Chap. $25 .$

储户在一段时间内将存款存入银行会获得利息，而贷方则为向银行的贷款支付利息。我们区分单利和复利，单利总是根据原始本金计算，而对于复利，利息是加到本金上的，因此下一个复利期的利息也会增加。例如，如果将 1000 欧元以每年 $10 \%$ 的利率存放在银行 2 年，那么它总共将赚取 200 欧元的单利。利息金额由下式计算
$$
\frac{1000 * 10 * 2}{100}=\text { 欧元 } 200 \text {. }
$$
计算本金 $P$、利率 $I$ 和时间 $T$（以年为单位：）的单利的一般公式是
$$
A=\frac{P \times I \times T}{100} 。
$$
从下面的例子可以看出，复利的计算更加复杂。
示例（复利）
计算所赚取的利息和新的本金将是 1000 欧元，该本金存放在银行，年利率为 10 美元 \%$ （复利），为期 3 年。
解决方案
在第 1 年结束时，赚取 100 欧元的利息，这将在第 2 年开始时资本化为新的本金 1100 欧元。在第 2 年末，获得 110 欧元的利息，使第 3 年年初的新本金为 1210 欧元。最后，在第 3 年末，又获得 121 欧元的利息，因此新的本金为 1331 欧元，3 年赚取的总利息是每年赚取的利息的总和（即 331 欧元）。这可以从表 $5.1 .$ 中看出
每年的新本金由 $a=1000$ 和 $r=10 / 100=0.1$ 的几何序列给出。
$$
1000,1000(1.1), 1000(1.1)^{2}, 1000(1.1)^{3}, \ldots
$$
94
5 序列、序列、排列和组合表 $5.1$ 计算复利复利
一般而言，若将投资本金$P$表示为比例，即$\left.r={ }^{R} / 100\right)$，则$$\mathrm{A}=P(1 +r)^{T} 。 $$
对于我们上面的例子，$A=1000, T=3$ and $r=0.1$ 因此，
$$
\开始{对齐}
一个 &=1000(1.1)^{3} \
&=1331(\text { 和以前一样 })
\end{对齐}
$$
复利公式的变体可以涵盖每年有 $m$ 复利期的情况，因此读者可以查阅可用的文本。我们将在第 1 章更详细地讨论基本金融数学。 25 美元。

图论代考

数学中使用的一些函数（或对象）（例如斐波那契数列）是
难以明确定义，最好由递归关系定义：即
递归定义一系列值的方程，一旦一个或多个初始值
值被定义。递归可用于定义函数、序列和
套。
递归定义有两个部分，即基本情况和
递归（归纳）步骤。基本情况通常定义函数的值
$n=0$ 或 $n=1$，而递归步骤指定如何应用
一个数的函数可以从其应用到一个或多个更小的函数中获得
数字。重要的是要注意递归定义，以确保它
不是循环的，也不会导致无限回归。函数的参数
递归步骤中定义的右侧通常小于
左侧的参数以确保终止（有一些不寻常的
递归定义的函数，例如 McCarthy 91 函数，其中 this 不是
案子）。当出现递归定义时，很自然地会问它是否意味着
任何东西，在某些情况下答案是否定的。不动点理论
为递归提供数学基础，并确保
功能/对象定义明确。
第 12 章（第 12.6 节）讨论了各种数学结构，例如偏
阶、完全偏阶和格，可用于给出
为递归奠定坚实的基础。给出了精确的数学含义
根据域和不动点理论递归定义的函数，它是
重要的是可以使用递归的条件
更多详细信息请参阅 [1]。
递归定义将包括至少一个非递归分支，每个递归分支都发生在与原始分支不同的上下文中，并带来
它更接近非递归情况。递归定义是一种强大而优雅的
给出语言结构的指称语义的方法。
接下来，我们给出阶乘函数 $\mathrm{~ N e x t i v i n g ~ d e n t i o n t}$ 的递归定义的例子
和斐波那契数。
例 4.4（函数的递归定义）阶乘函数 $n !$ 非常
在数学中很常见，其著名的定义是 $n !=$
$n(n-1)(n-2) \ldots 3.2 .1$ 和 $0 !=1$。根据基本情况的正式定义
归纳步骤如下：
$\begin{array}{ll}\text { 基本步骤 } & \text { fac }(0)=1 \ \text { 递归步骤 } & \text { fac }(n)=n * \operatorname{fac }(n-1)\end{数组}$
数学中使用的一些函数（或对象）（例如斐波那契数列）是
难以明确定义，最好由递归关系定义：即
递归定义一系列值的方程，一旦一个或多个初始值
值被定义。递归可用于定义函数、序列和
套。
递归定义有两个部分，即基本情况和
递归（归纳）步骤。基本情况通常定义函数的值
$n=0$ 或 $n=1$，而递归步骤指定如何应用
一个数的函数可以从其应用到一个或多个更小的函数中获得
数字。
重要的是要注意递归定义，以确保它
不是循环的，也不会导致无限回归。函数的参数
递归步骤中定义的右侧通常小于
左侧的参数以确保终止（有一些不寻常的
递归定义的函数，例如 $M c$ Carthy 91 函数，其中 this 不是
案子）。
当出现递归定义时，很自然地会问它是否意味着
任何东西，在某些情况下答案是否定的。不动点理论
为递归提供数学基础，并确保
功能/对象定义明确。
第 12 章（Sect. $12.6$）讨论了各种数学结构，例如偏
阶、完全偏阶和格，可用于给出
为递归奠定坚实的基础。给出了精确的数学含义
根据域和不动点理论递归定义的函数，它是
理解可以安全使用递归的条件至关重要。
$\mathrm{~ T h e ~ r e a d e r ~ i s ~ r e f e r}$
这个递归定义定义了一个数字的阶乘的过程
由基本情况确定，或由数字乘以的阶乘确定

数学代写| DISCRETE MATHEMATICS代考请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。

Cryptosystem
A system that describes how to encrypt or decrypt messages
Plaintext
Message in its original form
Ciphertext
Message in its encrypted form
Cryptographer
Invents encryption algorithms
Cryptanalyst
Breaks encryption algorithms or implementations

编码理论代写

编码理论（英语：Coding theory）是研究编码的性质以及它们在具体应用中的性能的理论。编码用于数据压缩、加密、纠错，最近也用于网络编码中。不同学科（如信息论、电机工程学、数学、语言学以及计算机科学）都研究编码是为了设计出高效、可靠的数据传输方法。这通常需要去除冗余并校正（或检测）数据传输中的错误。

编码共分四类：^[1]