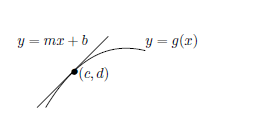$7.9$ Exercises
- Sally drives her Saturn over the 110 mile toll road in exactly $1.3$ hours. The speed limit on this toll road is 70 miles per hour and the fine for speeding is 10 dollars per mile per hour over the speed limit. How much should Sally pay?
- Two cars are careening down a freeway in Utah weaving in and out of traffic. Car A passes car B and then car B passes car A as the driver makes obscene gestures. This infuriates the driver of car A who passes car B while firing his handgun at the driver of car B. Show there are at least two times when both cars have the same speed. Then show there exists at least one time when they have the same acceleration. The acceleration is the derivative of the velocity.
- Show the cubic function $f(x)=5 x^{3}+7 x-18$ has only one real zero.
- Suppose $f(x)=x^{7}+|x|+x-12$. How many solutions are there to the equation, $f(x)=0$ ?
- Let $f(x)=|x-7|+(x-7)^{2}-2$ on the interval $[6,8]$. Then $f(6)=0=f(8)$. Does it follow from Rolle’s theorem that there exists $c \in(6,8)$ such that $f^{\prime}(c)=0$ ? Explain your answer.
- Suppose $f$ and $g$ are differentiable functions defined on $\mathbb{R}$. Suppose also that it is known that $\left|f^{\prime}(x)\right|>\left|g^{\prime}(x)\right|$ for all $x$ and that $\left|f^{\prime}(t)\right|>0$ for all $t$. Show that whenever $x \neq y$, it follows $|f(x)-f(y)|>|g(x)-g(y)|$. Hint: Use the Cauchy mean value theorem, Theorem 7.8.2.
- Show that, like continuous functions, functions which are derivatives have the intermediate value property. This means that if $f^{\prime}(a)<0<f^{\prime}(b)$ then there exists $x \in(a, b)$ such that $f^{\prime}(x)=0$. Hint: Argue the minimum value of $f$ occurs at an interior point of $[a, b]$.
- Find an example of a function which has a derivative at every point but such that the derivative is not everywhere continuous.
CHAPTER 7. THE DERIVATIVE
144 9. Consider the function $$ f(x) \equiv\left{\begin{array}{c}1 \text { if } x \geq 0 \ -1 \text { if } x<0\end{array}\right. $$
- Suppose $c \in I$, an open interval and that a function $f$, defined on $I$ has $n+1$ derivatives. Then for each $m \leq n$ the following formula holds for $x \in I .$
$$
f(x)=\sum_{k=0}^{m} f^{(k)}(c) \frac{(x-c)^{k}}{k !}+f^{(m+1)}(y) \frac{(x-c)^{m+1}}{(m+1) !}
$$
where $y$ is some point between $x$ and $c$. Fix $c, x$ in $I$. Let $K$ be a number, depending on $c, x$ such that
$$
f(x)-\left(f(c)+\sum_{k=1}^{n} \frac{f^{(k)}(c)}{k !}(x-c)^{k}+K(x-c)^{n+1}\right)=0
$$
Now the idea is to find $K$. To do this, let
$$
F(t)=f(x)-\left(f(t)+\sum_{k=1}^{n} \frac{f^{(k)}(t)}{k !}(x-t)^{k}+K(x-t)^{n+1}\right)
$$
Then $F(x)=F(c)=0$. Therefore, by Roll
e’s theorem there exists $y$ between $c$ and $x$ such that
$F^{\prime}(y)=0$. Do the differentiation and solve for $K$.
This is the main result on Taylor polynomials approximating a f
unction $f$. The term $f^{(m+1)}(y) \frac{(x-c)^{m+1}}{(m+1)
!}$ is called the Lagrange form of the remainder. - Let $f$ be a real continuous function defined on the interval $[0,1]$. Also suppose $f(0)=0$ and $f(1)=1$ and $f^{\prime}(t)$ exists for all $t \in(0,1)$. Show there exists $n$ distinct points $\left{s_{i}\right}_{i=1}^{n}$ of the interval such that
$$
\sum_{i=1}^{n} f^{\prime}\left(s_{i}\right)=n .
$$
Hint: Consider the mean value theorem applied to successive pairs in the following sum.
$$
f\left(\frac{1}{3}\right)-f(0)+f\left(\frac{2}{3}\right)-f\left(\frac{1}{3}\right)+f(1)-f\left(\frac{2}{3}\right)
$$ - Now suppose $f:[0,1] \rightarrow \mathbb{R}$ is continuous and differentiable on $(0,1)$ and $f(0)=0$ while $f(1)=1$. Show there are distinet points $\left{s_{i}\right}_{i=1}^{n} \subseteq(0,1)$ such that
$$
\sum_{i=1}^{n}\left(f^{\prime}\left(s_{i}\right)\right)^{-1}=n
$$
Hint: Let $0=t_{0}<t_{1}<\cdots<t_{n}=1$ and pick $x_{i} \in f^{-1}\left(t_{i}\right)$ such that these $x_{i}$ are increasing and $x_{n}=1, x_{0}=0$. Explain why you can do this. Then argue
$$
t_{i+1}-t_{i}=f\left(x_{i+1}\right)-f\left(x_{i}\right)=f^{\prime}\left(s_{i}\right)\left(x_{i+1}-x_{i}\right)
$$
and so
$$
\frac{x_{i+1}-x_{i}}{t_{i+1}-t_{i}}=\frac{1}{f^{\prime}\left(s_{i}\right)}
$$
Now choose the $t_{i}$ to be equally spaced. - Show that $(x+1)^{3 / 2}-x^{3 / 2}>2$ for all $x \geq 2$. Explain why for $n$ a natural number larger than or equal to 1 , there exists a natural number $m$ such that $(n+1)^{3}>m^{2}>n^{3}$. Hint: Verify directly for $n=1$ and use the above inequality to take care of the case where $n \geq 2$. This shows that between the cubes of any two natural numbers there is the square of a natural number.
莎莉驾驶她的土星在 110 英里的收费公路上行驶,时间正好是 1.3 美元。这条收费公路的限速是每小时 70 英里,超速的罚款是每小时每英里 10 美元。莎莉应该付多少钱?
两辆汽车在犹他州的高速公路上疾驰而过,进进出出。当司机做出猥亵手势时,A 车经过 B 车,然后 B 车经过 A 车。这激怒了 A 车的司机,他通过 B 车,同时向 B 车的司机开枪。表明至少有两次两辆车的速度相同。然后证明至少存在一次它们具有相同的加速度。加速度是速度的导数。
证明三次函数 $f(x)=5 x^{3}+7 x-18$ 只有一个实零。
4.假设$f(x)=x^{7}+|x|+x-12$。方程有多少个解,$f(x)=0$?
令 $f(x)=|x-7|+(x-7)^{2}-2$ 在区间 $[6,8]$ 上。那么$f(6)=0=f(8)$。从罗尔定理是否存在 $c \in(6,8)$ 使得 $f^{\prime}(c)=0$ ?解释你的答案。
假设 $f$ 和 $g$ 是定义在 $\mathbb{R}$ 上的可微函数。还假设已知 $\left|f^{\prime}(x)\right|>\left|g^{\prime}(x)\right|$ 对于所有 $x$ 并且 $\left |f^{\prime}(t)\right|>0$ 对于所有 $t$。证明只要 $x \neq y$,它遵循 $|f(x)-f(y)|>|g(x)-g(y)|$。提示:使用柯西中值定理,定理 7.8.2。
证明,像连续函数一样,导数函数也具有中间值属性。这意味着如果 $f^{\prime}(a)<0<f^{\prime}(b)$ 则存在 $x \in(a, b)$ 使得 $f^{\prime}( x)=0$。提示:论证 $f$ 的最小值出现在 $[a, b]$ 的内部点。
找一个函数的例子,它在每一点都有一个导数,但导数不是处处连续的。
第 7 章导数
144 9. 考虑函数 $$ f(x) \equiv\left{\begin{array}{c}1 \text { if } x \geq 0 \ -1 \text { if } x<0\end {数组}\对。 $$
假设$c \in I$,一个开区间,并且定义在$I$ 上的函数$f$ 有$n+1$ 个导数。然后对于每个 $m \leq n$,以下公式适用于 $x \in I .$
$$
f(x)=\sum_{k=0}^{m} f^{(k)}(c) \frac{(xc)^{k}}{k !}+f^{(m+1) }(y) \frac{(xc)^{m+1}}{(m+1) !}
$$
其中 $y$ 是 $x$ 和 $c$ 之间的某个点。修正 $I$ 中的 $c, x$。令 $K$ 是一个数字,取决于 $c, x$ 使得
$$
f(x)-\left(f(c)+\sum_{k=1}^{n} \frac{f^{(k)}(c)}{k !}(xc)^{k}+ K(xc)^{n+1}\right)=0
$$
现在的想法是找到$K$。为此,让
$$
F(t)=f(x)-\left(f(t)+\sum_{k=1}^{n} \frac{f^{(k)}(t)}{k !}(xt) ^{k}+K(xt)^{n+1}\right)
$$
那么$F(x)=F(c)=0$。因此,通过滚
e 的定理在 $c$ 和 $x$ 之间存在 $y$ 使得
$F^{\素数}(y)=0$。求微分并求解$K$。
这是近似 a f 的泰勒多项式的主要结果
涂抹$f$。项 $f^{(m+1)}(y) \frac{(x-c)^{m+1}}{(m+1)
!}$ 称为余数的拉格朗日形式。
令$f$ 为定义在区间$[0,1]$ 上的实数连续函数。还假设 $f(0)=0$ 和 $f(1)=1$ 和 $f^{\prime}(t)$ 对于所有 $t\in(0,1)$ 都存在。表明存在 $n$ 个不同的点 $\left{s_{i}\right}_{i=1}^{n}$ 使得
$$
\sum_{i=1}^{n} f^{\prime}\left(s_{i}\right)=n 。
$$
提示:考虑在以下总和中应用于连续对的平均值定理。
$$
f\left(\frac{1}{3}\right)-f(0)+f\left(\frac{2}{3}\right)-f\left(\frac{1}{3}\右)+f(1)-f\left(\frac{2}{3}\right)
$$
现在假设 $f:[0,1] \rightarrow \mathbb{R}$ 在 $(0,1)$ 和 $f(0)=0$ 上是连续且可微的,而 $f(1)=1$ .显示有不同点 $\left{s_{i}\right}_{i=1}^{n} \subseteq(0,1)$ 使得
$$
\sum_{i=1}^{n}\left(f^{\prime}\left(s_{i}\right)\right)^{-1}=n
$$
提示:令 $0=t_{0}<t_{1}<\cdots<t_{n}=1$ 并选择 $x_{i} \in f^{-1}\left(t_{i}\right) $ 使得这些 $x_{i}$ 增加并且 $x_{n}=1, x_{0}=0$。解释为什么你可以这样做。然后争论
$$
t_{i+1}-t_{i}=f\left(x_{i+1}\right)-f\left(x_{i}\right)=f^{\prime}\left(s_{i }\right)\left(x_{i+1}-x_{i}\right)
$$
所以
$$
\frac{x_{i+1}-x_{i}}{t_{i+1}-t_{i}}=\frac{1}{f^{\prime}\left(s_{i}\right )}
$$
现在选择 $t_{i}$ 等间距。
证明对于所有 $x \geq 2$,$(x+1)^{3 / 2}-x^{3 / 2}>2$。解释为什么对于大于或等于 1 的自然数 $n$ 存在一个自然数 $m$ 使得 $(n+1)^{3}>m^{2}>n^{3}$。提示:直接验证 $n=1$ 并使用上面的不等式来处理 $n \geq 2$ 的情况。这表明在任何两个自然数的立方之间都有一个自然数的平方。

微积分note Integer Multiples of Irrational Numbers 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。
代写
隐藏