如果你也在 怎样代写理论力学Theoretical Mechanics这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。理论力学Theoretical Mechanics是一组密切相关的经典力学的替代公式。它是由许多科学家和数学家在18世纪及以后,在牛顿力学之后发展起来的。由于牛顿力学考虑的是运动的矢量,特别是系统中各组成部分的加速度、动量、力,因此由牛顿定律和欧拉定律所支配的力学的另一个名称是矢量力学。
理论力学Theoretical Mechanics使用代表系统整体的运动标量属性–通常是其总动能和势能–而不是牛顿对单个粒子的矢量力。运动方程是由标量通过一些关于标量变化的基本原理推导出来的。分析力学使用代表系统整体的运动标量属性–通常是其总动能和势能–而不是牛顿对单个粒子的矢量力。运动方程是由标量通过一些关于标量变化的基本原理推导出来的。
my-assignmentexpert™ 理论力学Theoretical Mechanics作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的理论力学Theoretical Mechanics作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此理论力学Theoretical Mechanics作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在物理physics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的理论力学Theoretical Mechanics代写服务。我们的专家在物理physics代写方面经验极为丰富,各种理论力学Theoretical Mechanics相关的作业也就用不着 说。
我们提供的理论力学Theoretical Mechanics及其相关学科的代写,服务范围广, 其中包括但不限于:

物理代写|理论力学作业代写Theoretical Mechanics代考|Hamilton Function
We transform the Lagrangian,
$$
L=L\left(q_{1}, \ldots, q_{s}, \dot{q}{1}, \ldots, \dot{q}{s}, t\right),
$$
considering the generalized velocities $\dot{q}{1}, \ldots, \dot{q}{S}$ as active variables and replace them by the generalized momenta
$$
p_{i}=\frac{\partial L}{\partial \dot{q}{i}}, \quad i=1, \ldots, S $$ The Legendre-transform we got to know already in (1.169), except for the sign, as Hamilton function $$ H\left(q{1}, \ldots, q_{S}, p_{1}, \ldots, p_{S}, t\right)=\sum_{i=1}^{S} p_{i} \dot{q}{i}-L\left(q{1}, \ldots, q_{S}, \dot{q}{1}, \ldots, \dot{q}{S}, t\right)
$$
We have seen in Sect. 1.4.1 that there exists a close relationship between this function and the energy of the system. But before we come back to this point let us first derive the equations of motion which are connected to the Hamilton function $H$. For this purpose we build the total differential:
$$
\begin{aligned}
d H &=\sum_{i=1}^{S}\left(d p_{i} \dot{q}{i}+p{i} d \dot{q}{i}\right)-\sum{i=1}^{S}\left(\frac{\partial L}{\partial q_{i}} d q_{i}+\frac{\partial L}{\partial \dot{q}{i}} d \dot{q}{i}\right)-\frac{\partial L}{\partial t} d t \
&=\sum_{i=1}^{S}\left(\dot{q}{i} d p{i}-\frac{\partial L}{\partial q_{i}} d q_{i}\right)-\frac{\partial L}{\partial t} d t
\end{aligned}
$$
物理代写|理论力学作业代写Theoretical Mechanics代考|Simple Examples
The theory of the last section for the solution of problems in mechanics in the framework of the Hamiltonian formalism can be summarized by the following scheme:
- Selection of proper generalized coordinates:
$$
\mathbf{q} \equiv\left(q_{1}, q_{2}, \ldots, q_{S}\right)
$$ - Preparation of the transformation formulas:
$$
\begin{aligned}
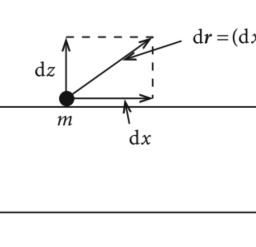
\mathbf{r}{i} &=\mathbf{r}{i}\left(q_{1}, \ldots, q_{S}, t\right), \
\dot{\mathbf{r}}{i} &=\dot{\mathbf{r}}{i}(\mathbf{q}, \dot{\mathbf{q}}, t)
\end{aligned}
$$ - Formulation of kinetic and potential energy as functions of the particle coordinates, then insertion of $2 .:$
$$
L(\mathbf{q}, \dot{\mathbf{q}}, t)=T(\mathbf{q}, \dot{\mathbf{q}}, t)-V(\mathbf{q}, t) \quad \text { (conservative system) }
$$ - Derivation of the generalized momenta:
$$
p_{j}=\frac{\partial L}{\partial \dot{q}{j}} \Longrightarrow p{j}=p_{j}(\mathbf{q}, \dot{\mathbf{q}}, t), \quad j=1,2, \ldots, S
$$ - Solving for $\dot{q}{j}$ : $$ \dot{q}{j}=\dot{q}_{j}(\mathbf{q}, \mathbf{p}, t), \quad j=1,2, \ldots, S
$$ - Lagrangian:
$$
L(\mathbf{q}, \dot{\mathbf{q}}(\mathbf{q}, \mathbf{p}, t), t)=\tilde{L}(\mathbf{q}, \mathbf{p}, t)
$$ - Legendre transformation:
$$
H(\mathbf{q}, \mathbf{p}, t)=\sum_{j=1}^{S} p_{j} \dot{q}_{j}(\mathbf{q}, \mathbf{p}, t)-\tilde{L}(\mathbf{q}, \mathbf{p}, t)
$$ - Formulation and integration of the canonical equations.For practicing the procedure let us derive according to this scheme the Hamilton function and its equations of motion for some rather simple examples.

理论力学代写
物理代写|理论力学作业代写THEORETICAL MECHANICS代考|HAMILTON FUNCTION
我们变换拉格朗日,
$$
L=L\left(q_{1}, \ldots, q_{s}, \dot{q}{1}, \ldots, \dot{q}{s}, t\right),
$$
considering the generalized velocities $\dot{q}{1}, \ldots, \dot{q}{S}$ as active variables and replace them by the generalized momenta
$$
p_{i}=\frac{\partial L}{\partial \dot{q}{i}}, \quad i=1, \ldots, S $$ The Legendre-transform we got to know already in (1.169), except for the sign, as Hamilton function $$ H\left(q{1}, \ldots, q_{S}, p_{1}, \ldots, p_{S}, t\right)=\sum_{i=1}^{S} p_{i} \dot{q}{i}-L\left(q{1}, \ldots, q_{S}, \dot{q}{1}, \ldots, \dot{q}{S}, t\right)
$$
We have seen in Sect. 1.4.1 that there exists a close relationship between this function and the energy of the system. But before we come back to this point let us first derive the equations of motion which are connected to the Hamilton function $H$. For this purpose we build the total differential:
$$
\begin{aligned}
d H &=\sum_{i=1}^{S}\left(d p_{i} \dot{q}{i}+p{i} d \dot{q}{i}\right)-\sum{i=1}^{S}\left(\frac{\partial L}{\partial q_{i}} d q_{i}+\frac{\partial L}{\partial \dot{q}{i}} d \dot{q}{i}\right)-\frac{\partial L}{\partial t} d t \
&=\sum_{i=1}^{S}\left(\dot{q}{i} d p{i}-\frac{\partial L}{\partial q_{i}} d q_{i}\right)-\frac{\partial L}{\partial t} d t
\end{aligned}
$$
物理代写|理论力学作业代写THEORETICAL MECHANICS代考|SIMPLE EXAMPLES
上一节在哈密顿形式主义框架下解决力学问题的理论可以概括为以下方案:
- 选择适当的广义坐标:
q≡(q1,q2,…,q小号) - 转换公式的准备:
$$
\begin{aligned}
\mathbf{r} {i} &=\mathbf{r} {i}\leftq_{1}, \ldots, q_{S}, t\rightq_{1}, \ldots, q_{S}, t\right, \
\dot{\mathbf{r}} {i} &=\dot{\mathbf{r}} {i}q,q˙,吨
\end{对齐}
$$ - 将动能和势能公式化为粒子坐标的函数,然后插入2.:
大号(q,q˙,吨)=吨(q,q˙,吨)−五(q,吨) (保守系统) - 广义动量的推导:
$$
p_{j}=\frac{\partial L}{\partial \dot{q} {j}} \Longrightarrow p {j}=p_{j}q,q˙,吨, \quad j=1,2, \ldots, S
$$ - 求解 $\dot{q} {j}:$ \dot{q} {j}=\dot{q}_{j}q,p,吨, \quad j=1,2, \ldots, S
$$ - 拉格朗日:
大号(q,q˙(q,p,吨),吨)=大号~(q,p,吨) - 传奇变身:
H(q,p,吨)=∑j=1小号pjq˙j(q,p,吨)−大号~(q,p,吨) - 规范方程的公式化和积分。为了练习这个过程,让我们根据这个方案推导出一些相当简单的例子的哈密尔顿函数及其运动方程。

物理代写|理论力学作业代写Theoretical Mechanics代考 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。