如果你也在 怎样代写博弈论Game theory 这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。博弈论Game theory在20世纪50年代被许多学者广泛地发展。它在20世纪70年代被明确地应用于进化论,尽管类似的发展至少可以追溯到20世纪30年代。博弈论已被广泛认为是许多领域的重要工具。截至2020年,随着诺贝尔经济学纪念奖被授予博弈理论家保罗-米尔格伦和罗伯特-B-威尔逊,已有15位博弈理论家获得了诺贝尔经济学奖。约翰-梅纳德-史密斯因其对进化博弈论的应用而被授予克拉福德奖。
博弈论Game theory是对理性主体之间战略互动的数学模型的研究。它在社会科学的所有领域,以及逻辑学、系统科学和计算机科学中都有应用。最初,它针对的是两人的零和博弈,其中每个参与者的收益或损失都与其他参与者的收益或损失完全平衡。在21世纪,博弈论适用于广泛的行为关系;它现在是人类、动物以及计算机的逻辑决策科学的一个总称。
同学们在留学期间,都对各式各样的作业考试很是头疼,如果你无从下手,不如考虑my-assignmentexpert™!
my-assignmentexpert™提供最专业的一站式服务:Essay代写,Dissertation代写,Assignment代写,Paper代写,Proposal代写,Proposal代写,Literature Review代写,Online Course,Exam代考等等。my-assignmentexpert™专注为留学生提供Essay代写服务,拥有各个专业的博硕教师团队帮您代写,免费修改及辅导,保证成果完成的效率和质量。同时有多家检测平台帐号,包括Turnitin高级账户,检测论文不会留痕,写好后检测修改,放心可靠,经得起任何考验!
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
我们在经济Economy代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的经济Economy代写服务。我们的专家在博弈论Game theory代写方面经验极为丰富,各种博弈论Game theory相关的作业也就用不着 说。
经济代写|博弈论代考Game theory代写|Kin selection
The animal kingdom overflows with examples of cooperation within the family. African hunting dogs regurgitate food to help out a hungry pack brother. Marmosets and tamarins help to care for their nephews and nieces in extended families. Male birds of some species do the same when their chances of being able to reproduce in the current year are low. Aphids give up their lives defending their siblings from attack. Musk oxen form a defensive ring around the weaker members of the family when attacked by wolves. Why is kinship so important in the animal kingdom?
Hamilton’s rule
Bill Hamilton’s Narrow Roads of Geneland is an account of the life and work of another oddball genius. He recently died a typically adventurous death on a field trip to Brazil. Hamilton deserves most of the credit for introducing game theory into biology, although I doubt that he ever heard of John Nash during the long years he strove, alone and unrecognized, to create a whole new field of research. One of his many achievements was to formulate the evolutionary explanation of cooperation within the family nowadays known as kin selection.
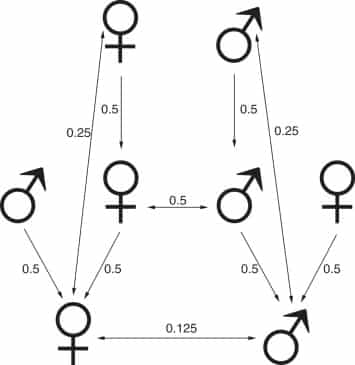
His point was famously anticipated in a semi-serious joke of J. B. S. Haldane. When asked whether he would give his life for another, he replied that the sacrifice would only be worthwhile if it saved two brothers or eight cousins! Haldane’s joke is only funny if you know that your degree of relationship to a full brother is one-half, and your degree of relationship to a full cousin is one-eighth.
It is sometimes said that the degree of relationship can’t really matter, because human beings share nearly all their genes anyway. But this is to miss the point that we are never concerned with genes that are always present in the human body, but only with a particular piece of behaviour that will be modified or left alone according to whether a recently mutated gene is present or absent.
Your degree of relationship to a relative is the probability that a recently mutated gene in your body is also in your relative’s body. To see that your degree of relationship to a cousin is one-eighth, imagine that your cousin is the daughter of your mother’s sister. The probability that a mutant gene in your body came from your mother rather than your father is one-half. If it came from your mother, the probability it is also in the body of your aunt is one-half. If it is in the body of your aunt, the probability she passed it to your cousin is one-half. Multiplying these three halves together, we get one-eighth.
What counts in calculating the fitness of a gene is the average number of times it gets replicated in the next generation. But it doesn’t matter which of two or more identical versions of a gene is copied. A copy made from a gene in my sister’s body is just as good as a copy made from an identical gene in my own body. When we figure out the fitness of a gene in my body, we therefore have to take account, not only of the effect of my behaviour on my own reproductive success, but of its effect on the reproductive success of my relatives. Hamilton called the outcome of such a calculation an inclusive fitness.
经济代写|博弈论代考Game theory代写|Social insects
A species is eusocial if it lives in colonies with overlapping generations in which one or a few individuals produce all the offspring, and the rest serve as sterile helpers. Eusociality is rare except among the Hymenoptera – the order of insects that includes ants, bees, and wasps. It used to be said that true eusociality has evolved independently at least 12 times in the Hymenoptera, but only twice elsewhere – the exceptional cases being the termites of the order Isoptera and the naked mole-rats of the order Rodentia. Later research has found other eusoscial species, the most interesting of which is a shrimp (Decapoda) that colonizes sponges on coral reefs. But the frequency of eusociality in the Hymenoptera remains a puzzle demanding an explanation.
Why did evolution generate casts of sterile workers? Why do they work tirelessly for the sake of others? Why is this phenomenon common among the Hymenoptera and rare elsewhere?
At one level, the puzzle is easy. Groups working together are usually more productive than individuals acting separately. In a beehive or an anthill, very large numbers of sterile workers specialize in protecting and caring for the young, while the queen specializes in being an egg-laying machine. As a consequence, the total number of young produced is immensely larger than if pairs of bees or ants brought up separate families by themselves.
It is clear why the queen benefits, but what’s in it for the workers? Each fertile child the queen produces is related to the workers. They are the workers’ brothers and sisters. A mutant gene that expresses itself in the body of a worker therefore has a lot of relatives to count when it computes its inclusive fitness. All the queen’s fertile children – weighted by their degree of relationship to a worker – must be counted when calculating the benefit to a worker of striving hard in support of the queen. The productivity of a beehive or an anthill then ensures that the balance comes down very firmly on the side of eusociality.
All this would be equally true of the human species if we had a sterile worker cast, but we traditionally bring up our children in extended families rather than biological factories. So why didn’t evolution take us down the same road as the Hymenoptera?
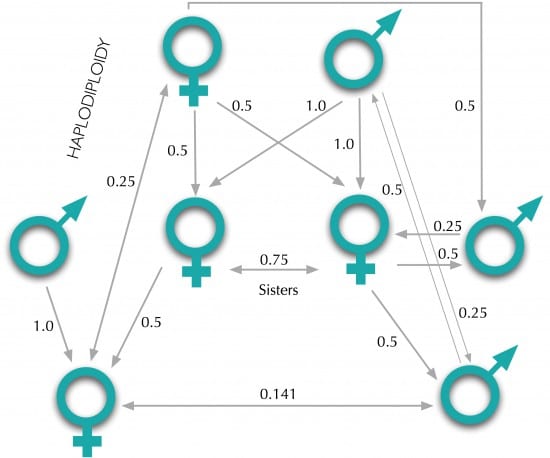
Bill Hamilton’s answer to this question depends on the fact that the Hymenoptera are haplodiploid, which means that unfertilized eggs grow into haploid males and fertilized eggs grow into diploid females. In a haploid species, each locus on a chromosome hosts just one gene. Humans are diploid, with each locus hosting two genes, one from the mother and one from the father. This is why the degree of relationship between human sisters is one-half, since a child gets one gene from each parent at every locus, and the gene it gets from each parent is equally likely to be either of the two genes the parent carries at that locus. By contrast, the degree of relationship between sisters in the Hymenoptera is three-quarters, because each locus on their chromosomes gets the same gene from their father, and a randomly chosen gene from the pair carried at that locus by their mother.
博弈论代写
经济代写|博弈论代考Game theory代写|Kin selection
动物王国里有很多家庭内部合作的例子。非洲猎犬反刍食物来帮助饥饿的同伴。狨猴和绢毛猴在大家庭中帮助照顾它们的侄子和侄女。当某些种类的雄鸟在当年繁殖的机会很低时,它们也会这样做。蚜虫为了保护自己的兄弟姐妹不受攻击而牺牲自己的生命。麝牛在受到狼的攻击时,会在较弱的家族成员周围形成防御圈。为什么亲缘关系在动物王国中如此重要?汉密尔顿的规则比尔·汉密尔顿的《吉兰狭窄的道路》是对另一位古怪天才的生活和工作的描述。他最近在去巴西的野外旅行中死于典型的冒险。汉密尔顿在将博弈论引入生物学方面的功劳最大,尽管我怀疑他是否听说过约翰·纳什,在他孤身一人、默默无闻地努力创造一个全新的研究领域的漫长岁月里。他的众多成就之一,是对现在被称为亲缘选择的家庭内部合作进行了进化解释。他的观点在J. B. S.霍尔丹的一个半严肃的笑话中被预料到了。当被问及是否愿意为他人牺牲生命时,他回答说,只有救了两个兄弟或八个表兄弟,这种牺牲才值得!霍尔丹的笑话只有在你知道你和一个同父异母的兄弟的亲缘关系是1 / 2,和一个同父异母的表兄的亲缘关系是1 / 8的情况下才会有趣。有时人们说,关系的程度并不重要,因为人类几乎所有的基因都是相同的。但这忽略了一点,即我们从不关心始终存在于人体内的基因,而只关心一种特定的行为,这种行为将根据最近突变的基因是否存在而被修改或不受影响。你与亲属的亲缘程度是指你体内最近发生突变的基因在你亲属体内发生突变的概率。要知道你和表亲的亲缘关系是八分之一,想象一下你的表亲是你母亲的姐姐的女儿。你体内的突变基因来自你母亲而不是父亲的概率是一半。如果它来自你的母亲,那么它也存在于你姑姑体内的概率是一半。如果它在你阿姨的身体里,她把它传给你表弟的概率是1 / 2。这三个1 / 2相乘,得到1 / 8。在计算一个基因的适合度时,重要的是它在下一代中被复制的平均次数。但是,复制两个或多个相同版本的基因中的哪一个并不重要。从我姐姐体内的基因复制出来的基因和从我自己体内的相同基因复制出来的基因一样好。因此,当我们计算出我体内某个基因的适合度时,我们不仅要考虑到我的行为对我自身繁殖成功的影响,还要考虑到它对我的亲属繁殖成功的影响。汉密尔顿把这种计算的结果称为“包容性适应度”
经济代写|博弈论代考Game theory代写|Social insects
如果一个物种生活在世代重叠的群体中,其中一个或几个个体产生所有的后代,而其余的个体充当不育的助手,那么这个物种就是群居的。除了膜翅目昆虫(包括蚂蚁、蜜蜂和黄蜂)之外,群居性很少见。过去人们常说,真正的群居性在膜翅目昆虫中至少独立进化了12次,但在其他地方只有两次——例外的例子是等翅目的白蚁和啮齿目的裸鼹鼠。后来的研究发现了其他的真社会性物种,其中最有趣的是一种虾(十足目),它在珊瑚礁的海绵上定居。但是膜翅目昆虫群居的频率仍然是一个需要解释的谜。
为什么进化会产生不育工人?为什么他们不知疲倦地为别人工作?为什么这种现象在膜翅目昆虫中很常见,而在其他地方却很少见?
在某种程度上,这个谜题很简单。团队合作通常比个人单独行动更有成效。在蜂巢或蚁丘中,大量的不育工蜂专门保护和照顾幼蜂,而蜂后则专门充当产卵机器。因此,产生的幼崽的总数要比一对蜜蜂或蚂蚁单独抚养一个家庭的数量大得多。
蜂王从中受益的原因很明显,但对工蚁有什么好处呢?蜂王生产的每一个可生育的孩子都与工蜂有关。他们是工人的兄弟姐妹。因此,在一个工人的身体中表达自己的突变基因,在计算其包容性适应度时,有很多亲戚要计算。在计算辛勤工作支持蚁后的工蜂的收益时,必须将蚁后所有可生育的子女——根据他们与工蜂的关系来加权。一个蜂巢或一个蚁丘的生产力确保了社会的平衡稳定地落在群居的一边。
如果我们有一个无菌的工人,这一切对人类来说也同样适用,但我们传统上是在大家庭中抚养我们的孩子,而不是在生物工厂里。那么为什么进化没有让我们走上膜翅目昆虫的道路呢?
比尔·汉密尔顿对这个问题的回答取决于膜翅目昆虫是单倍体的事实,这意味着未受精卵长成单倍体的雄性,受精卵长成二倍体的雌性。在单倍体物种中,染色体上的每个位点只承载一个基因。人类是二倍体,每个基因座有两个基因,一个来自母亲,一个来自父亲。这就是为什么人类姐妹之间的亲缘程度是二分之一,因为一个孩子在每个基因座上分别从父母那里得到一个基因,而从父母那里得到的基因同样有可能是父母在那个基因座上携带的两个基因中的一个。相比之下,膜翅目昆虫姐妹之间的亲缘程度是四分之三,因为她们染色体上的每个位点都从父亲那里得到了相同的基因,而在那个位点上随机选择的一个基因则由母亲携带。

经济代写|博弈论代考Game theory代写 请认准exambang™. exambang™为您的留学生涯保驾护航。
微观经济学代写
微观经济学是主流经济学的一个分支,研究个人和企业在做出有关稀缺资源分配的决策时的行为以及这些个人和企业之间的相互作用。my-assignmentexpert™ 为您的留学生涯保驾护航 在数学Mathematics作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的数学Mathematics代写服务。我们的专家在图论代写Graph Theory代写方面经验极为丰富,各种图论代写Graph Theory相关的作业也就用不着 说。
线性代数代写
线性代数是数学的一个分支,涉及线性方程,如:线性图,如:以及它们在向量空间和通过矩阵的表示。线性代数是几乎所有数学领域的核心。
博弈论代写
现代博弈论始于约翰-冯-诺伊曼(John von Neumann)提出的两人零和博弈中的混合策略均衡的观点及其证明。冯-诺依曼的原始证明使用了关于连续映射到紧凑凸集的布劳威尔定点定理,这成为博弈论和数学经济学的标准方法。在他的论文之后,1944年,他与奥斯卡-莫根斯特恩(Oskar Morgenstern)共同撰写了《游戏和经济行为理论》一书,该书考虑了几个参与者的合作游戏。这本书的第二版提供了预期效用的公理理论,使数理统计学家和经济学家能够处理不确定性下的决策。
微积分代写
微积分,最初被称为无穷小微积分或 “无穷小的微积分”,是对连续变化的数学研究,就像几何学是对形状的研究,而代数是对算术运算的概括研究一样。
它有两个主要分支,微分和积分;微分涉及瞬时变化率和曲线的斜率,而积分涉及数量的累积,以及曲线下或曲线之间的面积。这两个分支通过微积分的基本定理相互联系,它们利用了无限序列和无限级数收敛到一个明确定义的极限的基本概念 。
计量经济学代写
什么是计量经济学?
计量经济学是统计学和数学模型的定量应用,使用数据来发展理论或测试经济学中的现有假设,并根据历史数据预测未来趋势。它对现实世界的数据进行统计试验,然后将结果与被测试的理论进行比较和对比。
根据你是对测试现有理论感兴趣,还是对利用现有数据在这些观察的基础上提出新的假设感兴趣,计量经济学可以细分为两大类:理论和应用。那些经常从事这种实践的人通常被称为计量经济学家。
Matlab代写
MATLAB 是一种用于技术计算的高性能语言。它将计算、可视化和编程集成在一个易于使用的环境中,其中问题和解决方案以熟悉的数学符号表示。典型用途包括:数学和计算算法开发建模、仿真和原型制作数据分析、探索和可视化科学和工程图形应用程序开发,包括图形用户界面构建MATLAB 是一个交互式系统,其基本数据元素是一个不需要维度的数组。这使您可以解决许多技术计算问题,尤其是那些具有矩阵和向量公式的问题,而只需用 C 或 Fortran 等标量非交互式语言编写程序所需的时间的一小部分。MATLAB 名称代表矩阵实验室。MATLAB 最初的编写目的是提供对由 LINPACK 和 EISPACK 项目开发的矩阵软件的轻松访问,这两个项目共同代表了矩阵计算软件的最新技术。MATLAB 经过多年的发展,得到了许多用户的投入。在大学环境中,它是数学、工程和科学入门和高级课程的标准教学工具。在工业领域,MATLAB 是高效研究、开发和分析的首选工具。MATLAB 具有一系列称为工具箱的特定于应用程序的解决方案。对于大多数 MATLAB 用户来说非常重要,工具箱允许您学习和应用专业技术。工具箱是 MATLAB 函数(M 文件)的综合集合,可扩展 MATLAB 环境以解决特定类别的问题。可用工具箱的领域包括信号处理、控制系统、神经网络、模糊逻辑、小波、仿真等。