如果你也在 怎样代写现代代数Modern Algebra 这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。现代代数Modern Algebra就像数学的其他分支一样——只有从最基本的思想和例子中仔细地推导才能掌握。但这需要时间,而且有些目标在你实现之前是不明确的。
现代代数Modern Algebra这门学科的思想和方法几乎渗透到现代数学的每一个部分。此外,没有一门学科更适合培养处理抽象概念的能力,即理解和处理问题或学科的基本要素。这包括阅读数学的能力,提出正确的问题,解决问题,运用演绎推理,以及写出正确、切中要害、清晰的数学。
现代代数Modern Algebra代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。最高质量的现代代数Modern Algebra作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此现代代数Modern Algebra作业代写的价格不固定。通常在专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
同学们在留学期间,都对各式各样的作业考试很是头疼,如果你无从下手,不如考虑my-assignmentexpert™!
my-assignmentexpert™提供最专业的一站式服务:Essay代写,Dissertation代写,Assignment代写,Paper代写,Proposal代写,Proposal代写,Literature Review代写,Online Course,Exam代考等等。my-assignmentexpert™专注为留学生提供Essay代写服务,拥有各个专业的博硕教师团队帮您代写,免费修改及辅导,保证成果完成的效率和质量。同时有多家检测平台帐号,包括Turnitin高级账户,检测论文不会留痕,写好后检测修改,放心可靠,经得起任何考验!
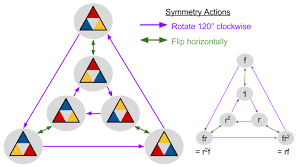
数学代写|现代代数代考Modern Algebra代写|Cauchy interpolation
The polynomial interpolation problem is, given a collection of sample values $v_i=$ $f\left(u_i\right) \in F$ for $0 \leq i<n$ of an unknown function $f: F \longrightarrow F$ at distinct points $u_0, \ldots, u_{n-1}$ of a field $F$, to compute a polynomial $g \in F[x]$ of degree less than $n$ that interpolates $g$ at those points, so that $g\left(u_i\right)=v_i$ for all $i$. We saw in Section 5.2 that such a polynomial always exists uniquely and learned how to compute it using the Lagrange interpolation formula.
A more general problem is Cauchy interpolation or rational interpolation, where furthermore $k \in{0, \ldots, n}$ is given and we are looking for a rational function $r / t \in F(x)$, with $r, t \in F[x]$, such that
$$
t\left(u_i\right) \neq 0 \text { and } \frac{r\left(u_i\right)}{t\left(u_i\right)}=v_i \text { for } 0 \leq i<n, \quad \operatorname{deg} r<k, \quad \operatorname{deg} t \leq n-k .
$$
Like polynomial interpolation, Cauchy interpolation can be used to approximate real-valued functions given only by their values at a finite set of points. Empirically, it is often the case that the approximation error is smaller for rational functions than for polynomials, in particular, when the function to be approximated has singularities; we will see an example below.
Obviously $t=1$ and $r=g$, where $g$ is an interpolating polynomial as above, is a solution to (20) for $k=n$, but it is not clear whether solutions for other values of $k$ exist. Multiplying (20) by $t\left(u_i\right)$ and dropping the requirement that it be nonzero, we obtain the weaker condition
$$
r\left(u_i\right)=t\left(u_i\right) v_i \text { for } 0 \leq i<n, \quad \operatorname{deg} r<k, \quad \operatorname{deg} t \leq n-k .
$$
Now for any $i, r\left(u_i\right)=t\left(u_i\right) v_i=t\left(u_i\right) g\left(u_i\right)$ if and only if $r \equiv \operatorname{tg} \bmod \left(x-u_i\right)$, and by the Chinese Remainder Theorem, Corollary 5.3, (21) is in turn equivalent to (17) with $m=\left(x-u_0\right) \cdots\left(x-u_{n-1}\right)$. The following consequence of Theorem 5.16 on rational function reconstruction gives a complete answer on existence and uniqueness of a solution to (20).
数学代写|现代代数代考Modern Algebra代写|Padé approximation
Let $F$ be a field and $g=\sum_{i \geq 0} g_i x^i \in F[[x]]$ with all $g_i \in F$ be a formal power series (Section 25.3). A Padé approximant to $g$ is a rational function $\rho=r / t \in F(x)$, with $r, t \in F[x]$ and $x \nmid t$, that “approximates” $g$ to a sufficiently high power of $x$. More precisely, $r / t$ is a $(k, n-k)$-Padé approximant to $g$ if
$$
x \nmid t \text { and } \frac{r}{t} \equiv g \bmod x^n, \quad \operatorname{deg} r<k, \quad \operatorname{deg} t \leq n-k ;
$$
the congruence is equivalent to $r \equiv \operatorname{tg} \bmod x^n$. Obviously $r=\sum_{0 \leq i<n} g_i x^i$, the Taylor expansion of order $n$ of $g$ around 0 , and $t=1$ is an (n,0)-Padé approximant for each $n \in \mathbb{N}$, but it is not clear whether approximants for $k<n$ exist. A more general question is to ask for Padé approximants around $u$ of a formal power series in $x-u$ for an arbitrary $u \in F$. This may be reduced to (22) by performing the shift of variable $x \longmapsto x+u$.
In numerical analysis, one is interested in approximating arbitrary (sufficiently smooth) real-valued functions by “simple” functions such as polynomials or rational functions. Taylor expansions and Padé approximants provide such approximations in the vicinity of the origin (or any other point, after an appropriate change of variable). As in the case of interpolation, it was observed empirically that sometimes rational functions yield a much smaller approximation error, in particular when the function to be approximated has singularities; see Example 5.23 below.
The similarity with Cauchy interpolation is clear: instead of prescribing the values of $\rho$ at $n$ distinct points $u_0, \ldots, u_{n-1}$, we have $u_0=\cdots=u_{n-1}=0$ and prescribe an initial segment of the Taylor expansion of $\rho$ at $u_0$. Indeed the statements of the previous section carry over almost literally if we replace $m=\left(x-u_0\right) \cdots\left(x-u_{n-1}\right)$ by $m=x^n$. The following is a consequence of Theorem 5.16.
现代代数代写
数学代写|现代代数代考Modern Algebra代写|Cauchy interpolation
多项式插值问题是,给定一个未知函数$f: F \longrightarrow F$在不同点上的采样值$v_i=$$f\left(u_i\right) \in F$对于$0 \leq i<n$的采样值集合$u_0, \ldots, u_{n-1}$对于一个字段$F$,计算一个次数小于$n$的多项式$g \in F[x]$在这些点上插值$g$,使得$g\left(u_i\right)=v_i$对于所有$i$。我们在5.2节中看到,这样的多项式总是唯一存在的,并学习了如何使用拉格朗日插值公式来计算它。
一个更普遍的问题是柯西插值或有理插值,其中更进一步$k \in{0, \ldots, n}$是给定的,我们正在寻找一个有理函数$r / t \in F(x)$,用$r, t \in F[x]$,这样
$$
t\left(u_i\right) \neq 0 \text { and } \frac{r\left(u_i\right)}{t\left(u_i\right)}=v_i \text { for } 0 \leq i<n, \quad \operatorname{deg} r<k, \quad \operatorname{deg} t \leq n-k .
$$
与多项式插值一样,柯西插值也可以用来逼近实值函数,而实值函数只能由它们在有限点上的值给出。根据经验,通常有理函数的近似误差比多项式的近似误差小,特别是当要近似的函数具有奇点时;我们将在下面看到一个示例。
显然$t=1$和$r=g$,其中$g$是如上所述的插值多项式,是$k=n$的(20)的解,但不清楚$k$的其他值是否存在解。将(20)乘以$t\left(u_i\right)$并去掉非零的要求,我们得到较弱的条件
$$
r\left(u_i\right)=t\left(u_i\right) v_i \text { for } 0 \leq i<n, \quad \operatorname{deg} r<k, \quad \operatorname{deg} t \leq n-k .
$$
现在对于任何$i, r\left(u_i\right)=t\left(u_i\right) v_i=t\left(u_i\right) g\left(u_i\right)$当且仅当$r \equiv \operatorname{tg} \bmod \left(x-u_i\right)$,根据中国剩余定理,推论5.3,(21)又与$m=\left(x-u_0\right) \cdots\left(x-u_{n-1}\right)$等价于(17)。定理5.16关于有理函数重构的结论给出了(20)解的存在唯一性的完整答案。
数学代写|现代代数代考Modern Algebra代写|Padé approximation
让 $F$ 成为一个领域 $g=\sum_{i \geq 0} g_i x^i \in F[[x]]$ 与所有人 $g_i \in F$ 是一个正式的幂级数(第25.3节)。大约是 $g$ 是一个有理函数 $\rho=r / t \in F(x)$, with $r, t \in F[x]$ 和 $x \nmid t$,即“近似”。 $g$ 达到足够高的功率 $x$. 更准确地说, $r / t$ 是? $(k, n-k)$-近似于 $g$ 如果
$$
x \nmid t \text { and } \frac{r}{t} \equiv g \bmod x^n, \quad \operatorname{deg} r近似值 $u$ 的形式幂级数 $x-u$ 对于任意的 $u \in F$. 这可以通过执行变量移位减少到(22) $x \longmapsto x+u$.
在数值分析中,人们感兴趣的是用多项式或有理函数等“简单”函数逼近任意(足够光滑的)实值函数。泰勒展开和帕德帕尔近似在原点附近(或任何其他点,在适当改变变量后)提供了这样的近似。正如在插值的情况下,经验观察到,有时有理函数产生的近似误差要小得多,特别是当要近似的函数具有奇点时;参见下面的例5.23。
与柯西插值的相似之处很明显:我们有$u_0=\cdots=u_{n-1}=0$,并规定了$\rho$在$u_0$的泰勒展开的初始段,而不是规定$\rho$在$n$不同点$u_0, \ldots, u_{n-1}$的值。实际上,如果我们将$m=\left(x-u_0\right) \cdots\left(x-u_{n-1}\right)$替换为$m=x^n$,上一节的语句几乎可以逐字保留。下面是定理5.16的一个推论。

数学代写|现代代数代考Modern Algebra代写 请认准exambang™. exambang™为您的留学生涯保驾护航。