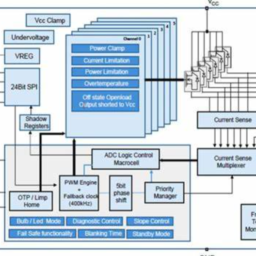
4.8.1 Sequential Compactness
First I will discuss the very important concept of sequential compactness. This is a property that some sets have. A set of numbers is sequentially compact if every sequence contained in the set has a subsequence which converges to a point in the set. It is unbelievably useful whenever you try to understand existence theorems.
Definition 4.8.1 A set, $K \subseteq \mathbb{F}^{p}$ is sequentially compact if whenever $\left{a_{n}\right} \subseteq K$ is a sequence, there exists a subsequence, $\left{a_{n_{k}}\right}$ such that this subsequence converges to a point of $K$.
The following theorem is part of the Heine Borel theorem.
Theorem 4.8.2 Every closed interval $[a, b]$ is sequentially compact.
Proof: Let $\left{x_{n}\right} \subseteq[a, b] \equiv I_{0}$. Consider the two intervals $\left[a, \frac{a+b}{2}\right]$ and $\left[\frac{a+b}{2}, b\right]$ each of which has length $(b-a) / 2$. At least one of these intervals contains $I_{n}$ for infinitely many values of $n$. Call this interval $I_{1}$. Now do for $I_{1}$ what was done for $I_{0}$. Split it in half and let $I_{2}$ be the interval which contains $I_{n}$ for infinitely many values of $n$. Continue this way obtaining a sequence of nested intervals $I_{0} \supseteq I_{1} \supseteq I_{2} \geq I_{3} \cdots$ where the length of $I_{n}$ is $(b-a) / 2^{n}$. Now pick $n_{1}$ such that $x_{n_{1}} \in I_{1}, n_{2}$ such that $n_{2}>n_{1}$ and $x_{n_{2}} \in I_{2}, n_{3}$ such that $n_{3}>n_{2}$ and $x_{n_{1}} \in I_{3}$, etc. (This can be done because in each case the intervals contained $x_{n}$ for infinitely many values of $n_{-}$) By the nested interval lemms there exists a point $c$ contained in all these intervals. Furthermore, $\left|x_{n_{k}}-c\right|<(b-a) 2^{-k}$ and so $\lim {k \rightarrow \infty} x{n_{k}}=c \in[a, b] .$ Corollary 4.8.3 $R \equiv \prod_{k-1}^{p}\left[a_{k}, b_{k}\right]$ is sequentially compact in $\mathbb{R}^{p} .$ Proof: Let $\left{x_{n}\right}_{n-1}^{\infty} \subseteq R, x_{n}=\left(x_{1}^{n}, \cdots, x_{p}^{n}\right)$. Then there is a subsequence, still denoted with $n$ such that $\left{x_{1}^{n}\right}$ converges to a point $I_{1} \in\left[a_{1}, b_{1}\right]$. Now there exists a further
subsequence, still denoted with $n$ such that $x_{2}^{n}$ converges to $x_{2} \in\left[a_{2}, b_{2}\right]$. Continuing to take subsequence, there is a subsequence, still denoted with $n$ such that $x_{k}^{n} \rightarrow x_{k} \in\left[a_{k}, b_{k}\right]$ for each $k$. By Proposition 4.4.3, this shows that this subsequence converges to $x=\left(x_{1}, \cdots, x_{p}\right)$. 4.8.2 Closed and Open Sets I have boen using the terminology $[a, b]$ is a closed interval to mean it is an interval which contains the two endpoints. However, there is a more general notion of what it means to be closed. Similarly there is a general notion of what it means to be open. Definition $4.8 .4$ Let $U$ be a set of points in $\mathrm{F}^{p} . A$ point $p \in U$ is said to be an interior point if whenever $|x-p|$ is sufficiently small, it follows $x \in U$ also. The set of points, $x$ which are closer to $p$ than $\delta$ is denoted by $$ B(p, \delta) \equiv{x \in \mathbb{F}:|x-p|<\delta} . $$ This symbol, $B(p, \delta)$ is called an open ball of radius $\delta$. Thus a point $p$ is an interior point of $U$ if there exists $\delta>0$ such that $p \in B(p, \delta) \subseteq U$. An open set is one for which every point of the set is an interior point. Closed sets are those which are complements of open sets. Thus $H$ is closed means $H^{C}$ is open.
What is an example of an open set? The simplest example is an open ball.
Proposition $4.8 .5 B(p, \delta)$ is an open set and $D(p, r) \equiv{x:|x-p| \leq r}$ is a closed set.
Proof: It is necessary to show every point is an interior point. Let $x \in B(p, \delta)$. Then let $r=\delta-|x-p|$. It follows $r>0$ because it is given that $|x-p|<\delta$. Now consider $z \in B(x, r)$. From Proposition 4.4.2 the triangle inequality,
$$
|z-p| \leq|z-x|+|x-p|<r+|x-p|=\delta-|x-p|+|x-p|=\delta
$$
and so $z \in B(p, \delta)$. That is $B(x, r) \subseteq B(p, \delta)$. Since $x$ was arbitrary, this has shown every point of the ball is an interior point. Thus the ball is an open set.
Consider the last assertion. If $y \notin D(p, r)$, then $|y-p|>r$ and you could consider $B(y,|y-p|-r)$. If $z \in B(y,|y-p|-r)$, then
$$
\begin{aligned}
|z-p| &=|z-y+y-p| \geq|y-p|-|z-y| \
&>|y-p|-(|y-p|-r)=r
\end{aligned}
$$
and so $z \notin D(p, r)$ which shows that the complement of $D(p, r)$ is open so this set is closed.
微积分note The Nested Interval Lemma
4.8.1 顺序紧凑性
首先,我将讨论非常重要的顺序紧凑性概念。这是某些集合具有的属性。如果集合中包含的每个序列都有一个收敛到集合中一个点的子序列,则一组数字是顺序紧致的。每当您尝试理解存在定理时,它都会非常有用。
定义 4.8.1 一个集合 $K \subseteq \mathbb{F}^{p}$ 是顺序紧的,如果当 $\left{a_{n}\right} \subseteq K$ 是一个序列时,存在一个子序列,$\left{a_{n_{k}}\right}$ 使得这个子序列收敛到 $K$ 的点。
以下定理是 Heine Borel 定理的一部分。
定理 4.8.2 每个闭区间 $[a, b]$ 是顺序紧的。
证明:设$\left{x_{n}\right} \subseteq[a, b] \equiv I_{0}$。考虑两个区间 $\left[a, \frac{a+b}{2}\right]$ 和 $\left[\frac{a+b}{2}, b\right]$ 每个区间都有长度$(ba) / 2$。这些区间中的至少一个包含 $I_{n}$ 用于无限多个 $n$ 值。将此区间称为 $I_{1}$。现在为 $I_{1}$ 做为 $I_{0}$ 所做的事情。把它分成两半,让 $I_{2}$ 是包含 $I_{n}$ 的区间,对于无限多的 $n$ 值。继续以这种方式获得一系列嵌套区间 $I_{0} \supseteq I_{1} \supseteq I_{2} \geq I_{3} \cdots$ 其中 $I_{n}$ 的长度为 $(ba) / 2^{n}$。现在选择 $n_{1}$ 使得 $x_{n_{1}} \in I_{1}, n_{2}$ 使得 $n_{2}>n_{1}$ 和 $x_{n_{2 }} \in I_{2}, n_{3}$ 使得 $n_{3}>n_{2}$ 和 $x_{n_{1}} \in I_{3}$ 等(这可以是之所以这样做,是因为在每种情况下,区间都包含无限多个 $n_{-}$ 值的 $x_{n}$) 通过嵌套区间 lemms,存在一个包含在所有这些区间中的点 $c$。此外,$\left|x_{n_{k}}-c\right|<(ba) 2^{-k}$ 等 $\lim {k \rightarrow \infty} x{n_{k}}= c \in[a, b] .$ 推论 4.8.3 $R \equiv \prod_{k-1}^{p}\left[a_{k}, b_{k}\right]$ 在 $\mathbb{R}^{p} 中是顺序紧致的。 $ 证明:设 $\left{x_{n}\right}_{n-1}^{\infty} \subseteq R, x_{n}=\left(x_{1}^{n}, \cdots , x_{p}^{n}\right)$.然后有一个子序列,仍然用 $n$ 表示,使得 $\left{x_{1}^{n}\right}$ 收敛到点 $I_{1} \in\left[a_{1} , b_{1}\right]$。现在存在另一个子序列,仍然用 $n$ 表示,使得 $x_{2}^{n}$ 收敛到 $x_{2} \in\left[a_{2}, b_{2}\right]$ .继续取子序列,有一个子序列,仍然用 $n$ 表示,这样 $x_{k}^{n} \rightarrow x_{k} \in\left[a_{k}, b_{k}\right]每$k$$。根据命题 4.4.3,这表明这个子序列收敛到 $x=\left(x_{1}, \cdots, x_{p}\right)$。 4.8.2 闭集和开集 我已经使用术语 $[a, b]$ 是一个封闭区间来表示它是一个包含两个端点的区间。但是,对于关闭的含义,有一个更一般的概念。同样,对于开放意味着什么,也有一个普遍的概念。 定义 $4.8 .4$ 令 $U$ 为 $\mathrm{F}^{p} 中的一组点。如果每当 $|x-p|$ 足够小,它也跟随在 $x\in U$ 之后,则称 A$ 点 $p \in U$ 是一个内点。比 $\delta$ 更接近 $p$ 的点集 $x$ 表示为 $$ B(p, \delta) \equiv{x \in \mathbb{F}:|x-p|<\delta} 。 $$ 这个符号 $B(p, \delta)$ 被称为半径为 $\delta$ 的开球。因此,如果存在 $\delta>0$ 使得 $p \in B(p, \delta) \subseteq U$,则点 $p$ 是 $U$ 的内点。开集是一个集合的每个点都是一个内点的集合。闭集是开集的补集。因此 $H$ 关闭意味着 $H^{C}$ 是打开的。
什么是开集的例子?最简单的例子是空位球。
命题 $4.8 .5 B(p, \delta)$ 是一个开集并且 $D(p, r) \equiv{x:|x-p| \leq r}$ 是一个闭集。
证明:有必要证明每个点都是一个内点。设 $x \in B(p, \delta)$。然后令$r=\delta-|x-p|$。它遵循$r>0$,因为给定$|x-p|<\delta$。现在考虑 $z \in B(x, r)$。从命题 4.4.2 三角不等式,
$$
|z-p| \leq|z-x|+|x-p|<r+|x-p|=\delta-|x-p|+|x-p|=\delta
$$
所以 $z \in B(p, \delta)$。即$B(x, r) \subseteq B(p, \delta)$。由于 $x$ 是任意的,这表明球的每个点都是一个内部点。因此球是一个开集。
考虑最后一个断言。如果$y \notin D(p, r)$,那么$|y-p|>r$,你可以考虑$B(y,|y-p|-r)$。如果$z \in B(y,|y-p|-r)$,那么
$$
\开始{对齐}
|z-p| &=|z-y+y-p| \geq|y-p|-|z-y| \
&>|y-p|-(|y-p|-r)=r
\end{对齐}
$$
所以 $z \notin D(p, r)$ 这表明 $D(p, r)$ 的补集是开的,所以这个集合是闭的

微积分note Integer Multiples of Irrational Numbers 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。