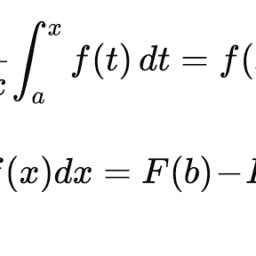
It is desirable to be able to differentiate and multiply power series. Recall
$$
f^{\prime}(x) \equiv \lim _{h \rightarrow 0} \frac{f(x+h)-f(x)}{h}
$$
Here $h, x, f$ all can have values in $\mathbb{C}$. The following theorem says you can differentiate power series in the most natural way on the disk of convergence, just as you would differentiate a polynomial. This theorem may seem obvious, but it is a serious mistake to think this. You usually cannot differentiate an infinite series whose terms are functions even if the functions are themselves polynomials. The following is special and pertains to power series. It is another example of the interchange of two limits, in this case, the limit involved in taking the derivative and the limit of the sequence of finite sums.
When you formally differentiate a series term by term, the result is called the derived series.
Theorem 8.2.1 Let $\sum_{n=0}^{\infty} a_{n}(x-a)^{n}$ be a Taylor series having radius of convergence $R>0$ and let
$$
f(x) \equiv \sum_{n=0}^{\infty} a_{n}(x-a)^{n}
$$
for $|x-a|<R$. Then
$$
f^{\prime}(x)=\sum_{n=0}^{\infty} a_{n} n(x-a)^{n-1}=\sum_{n=1}^{\infty} a_{n} n(x-a)^{n-1}
$$
and this new differentiated power series, the derived series, has radius of convergence equal to R. Also, $f(x)$ given by the Taylor series is infinitely differentiable on the interior of its disk of convergence.
Proof: First consider the claim that the derived series has radius of convergence equal to $R$. Let $\hat{R}$ be the radius of convergence of the derived series. Then from Proposition $4.10 .13$ and Lemma 8.1.2.
$$
\frac{1}{\hat{R}} \equiv \lim \sup {n \rightarrow \infty}\left|a{n}\right|^{1 / n} n^{1 / n}=\lim \sup {n \rightarrow \infty}\left|a{n}\right|^{1 / n} \equiv \frac{1}{R}
$$
and so $\hat{R}=R$. If $\limsup {n \rightarrow \infty}\left|a{n}\right|^{1 / n}=0$, the same is true of $\limsup {n \rightarrow \infty}\left|a{n}\right|^{1 / n} n^{1 / n}$ and in this case, the series and derived series both have radius of convergence equal to $\infty$.
Now let $r<R$, the radius of convergence of both series, and suppose $|x-a|<r$. Let $\delta$ be small enough that if $|h|<\delta$, then
$$
|x+h-a| \leq|x-a|+|h|<r
$$
also. Thus, $\lim \sup {k \rightarrow \infty}\left|a{k}\right|^{1 / k} r<1$.
Then for $|h|<\delta$, consider the difference quotient.
$$
\frac{f(x+h)-f(x)}{h}=\frac{1}{h} \sum_{k=0}^{\infty} a_{k}\left((x+h-a)^{k}-(x-a)^{k}\right)
$$Using the binomial theorem,
$$
\begin{aligned}
\frac{f(x+h)-f(x)}{h} &=\frac{1}{h} \sum_{k=0}^{\infty} a_{k}\left((x+h-a)^{k}-(x-a)^{k}\right) \
&=\frac{1}{h} \sum_{k=1}^{\infty} a_{k}\left(\sum_{j=0}^{k}\left(\begin{array}{c}
k \
j
\end{array}\right)(x-a)^{j} h^{k-j}-(x-a)^{k}\right) \
&=\sum_{k=1}^{\infty} a_{k}\left(\sum_{j=0}^{k-1}\left(\begin{array}{c}
k \
j
\end{array}\right)(x-a)^{j} h^{(k-1)-j}\right)
\end{aligned}
$$
Then
$$
\begin{aligned}
&\left|\frac{f(x+h)-f(x)}{h}-\sum_{k=1}^{\infty} a_{k} k(x-a)^{k-1}\right| \
=&\left|\sum_{k=1}^{\infty} a_{k}\left(\sum_{j=0}^{k-1}\left(\begin{array}{c}
k \
j
\end{array}\right)(x-a)^{j} h^{(k-1)-j}-k(x-a)^{k-1}\right)\right| \
=&\left|\sum_{k=2}^{\infty} a_{k}\left(\sum_{j=0}^{k-1}\left(\begin{array}{c}
k \
j
\end{array}\right)(x-a)^{j} h^{(k-1)-j}-k(x-a)^{k-1}\right)\right| \
=&\left|\sum_{k=2}^{\infty} a_{k}\left(\sum_{j=0}^{k-2}\left(\begin{array}{c}
k \
j
\end{array}\right)(x-a)^{j} h^{(k-1)-j}\right)\right|
\end{aligned}
$$
Therefore,
$$
\left|\frac{f(x+h)-f(x)}{h}-\sum_{k=1}^{\infty} a_{k} k(x-a)^{k-1}\right| \leq \sum_{k=2}^{\infty}\left|a_{k}\right|\left(\sum_{j=0}^{k-2}\left(\begin{array}{c}
k \
j
\end{array}\right)|x-a|^{j}|h|^{(k-1)-j}\right)
$$
Now it is clear that $k(k-1)\left(\begin{array}{c}k-2 \ j\end{array}\right) \geq\left(\begin{array}{l}k \ j\end{array}\right)$ and so
$$
\begin{aligned}
&=|h| \sum_{k=2}^{\infty}\left|a_{k}\right|\left(\sum_{j=0}^{k-2}\left(\begin{array}{c}
k \
j
\end{array}\right)|x-a|^{j}|h|^{(k-2)-j}\right) \
&\leq|h| \sum_{k=2}^{\infty}\left|a_{k}\right| k(k-1) \sum_{j=0}^{k-2}\left(\begin{array}{c}
k-2 \
j
\end{array}\right)|x-a|^{j}|h|^{(k-2)-j}
\end{aligned}
$$
$=|h| \sum_{k=2}^{\infty}\left|a_{k}\right| k(k-1)(|x-a|+|h|)^{k-2}<|h| \sum_{k=2}^{\infty}\left|a_{k}\right| k(k-1)$ Byrassumption and what was just observed about $\lim {k \rightarrow \infty} k^{1 / k}$ By assumption and what was just observed about $\lim {k \rightarrow \infty} k^{1 / k}$, $$ \lim \sup {k \rightarrow \infty}\left(\left|a{k}\right| k(k-1) r^{k-2}\right)^{1 / k}<1 $$
$$
\left|\frac{f(x+h)-f(x)}{h}-\sum_{k=1}^{\infty} a_{k} k(x-a)^{k-1}\right|<C|h|
$$
希望能够区分和乘幂级数。记起
$$
f^{\prime}(x) \equiv \lim _{h \rightarrow 0} \frac{f(x+h)-f(x)}{h}
$$
这里 $h, x, f$ 都可以有 $\mathbb{C}$ 中的值。下面的定理说你可以在收敛盘上以最自然的方式微分幂级数,就像你微分多项式一样。这个定理似乎很明显,但这样想是一个严重的错误。即使函数本身是多项式,您通常也无法区分其项是函数的无限级数。以下是特殊的,属于幂级数。这是两个极限互换的另一个例子,在这种情况下,取导数所涉及的极限和有限和序列的极限。
当您正式区分一个系列术语时,结果称为派生系列。
定理 8.2.1 令 $\sum_{n=0}^{\infty} a_{n}(x-a)^{n}$ 是一个泰勒级数,其收敛半径为 $R>0$,并令
$$
f(x) \equiv \sum_{n=0}^{\infty} a_{n}(x-a)^{n}
$$
对于 $|x-a|<R$。然后
$$
f^{\prime}(x)=\sum_{n=0}^{\infty} a_{n} n(xa)^{n-1}=\sum_{n=1}^{\infty} a_ {n} n(xa)^{n-1}
$$
而这个新的微分幂级数,即派生级数,其收敛半径等于 R。此外,泰勒级数给出的 $f(x)$ 在其收敛圆盘的内部是无限可微的。
证明:首先考虑派生级数的收敛半径等于$R$。令 $\hat{R}$ 为派生级数的收敛半径。然后来自命题 $4.10 .13$ 和引理 8.1.2。
$$
\frac{1}{\hat{R}} \equiv \lim \sup {n \rightarrow \infty}\left|a{n}\right|^{1 / n} n^{1 / n}= \lim \sup {n \rightarrow \infty}\left|a{n}\right|^{1 / n} \equiv \frac{1}{R}
$$
所以$\hat{R}=R$。如果$\limsup {n \rightarrow \infty}\left|a{n}\right|^{1 / n}=0$,同样适用于$\limsup_{n \rightarrow \infty}\left |a_{n}\right|^{1 / n} n^{1 / n}$ 并且在这种情况下,级数和派生级数的收敛半径都等于 $\infty$。
现在让$r<R$,两个级数的收敛半径,假设$|x-a|<r$。令 $\delta$ 足够小,如果 $|h|<\delta$,那么
$$
|x+h-a| \leq|x-a|+|h|<r
$$
还。因此,$\lim \sup_{k \rightarrow \infty}\left|a_{k}\right|^{1 / k} r<1$。
然后对于 $|h|<\delta$,考虑差商。
$$
\frac{f(x+h)-f(x)}{h}=\frac{1}{h} \sum_{k=0}^{\infty} a_{k}\left((x+ha )^{k}-(xa)^{k}\right)
$$使用二项式定理,
$$
\开始{对齐}
\frac{f(x+h)-f(x)}{h} &=\frac{1}{h} \sum_{k=0}^{\infty} a_{k}\left((x+ ha)^{k}-(xa)^{k}\right) \
&=\frac{1}{h} \sum_{k=1}^{\infty} a_{k}\left(\sum_{j=0}^{k}\left(\begin{array}{c }
k \
j
\end{数组}\right)(x-a)^{j} h^{k-j}-(x-a)^{k}\right) \
&=\sum_{k=1}^{\infty} a_{k}\left(\sum_{j=0}^{k-1}\left(\begin{array}{c}
k \
j
\end{数组}\right)(x-a)^{j} h^{(k-1)-j}\right)
\end{对齐}
$$
然后
$$
\开始{对齐}
&\left|\frac{f(x+h)-f(x)}{h}-\sum_{k=1}^{\infty} a_{k} k(xa)^{k-1}\对| \
=&\left|\sum_{k=1}^{\infty} a_{k}\left(\sum_{j=0}^{k-1}\left(\begin{array}{c}
k \
j
\end{数组}\right)(x-a)^{j} h^{(k-1)-j}-k(x-a)^{k-1}\right)\right| \
=&\left|\sum_{k=2}^{\infty} a_{k}\left(\sum_{j=0}^{k-1}\left(\begin{array}{c}
k \
j
\end{数组}\right)(x-a)^{j} h^{(k-1)-j}-k(x-a)^{k-1}\right)\right| \
=&\left|\sum_{k=2}^{\infty} a_{k}\left(\sum_{j=0}^{k-2}\left(\begin{array}{c}
k \
j
\end{数组}\right)(x-a)^{j} h^{(k-1)-j}\right)\right|
\end{对齐}
$$
所以,
$$
\left|\frac{f(x+h)-f(x)}{h}-\sum_{k=1}^{\infty} a_{k} k(xa)^{k-1}\right | \leq \sum_{k=2}^{\infty}\left|a_{k}\right|\left(\sum_{j=0}^{k-2}\left(\begin{array}{c }
k \
j
\end{数组}\right)|x-a|^{j}|h|^{(k-1)-j}\right)
$$
现在很清楚 $k(k-1)\left(\begin{array}{c}k-2 \ j\end{array}\right) \geq\left(\begin{array}{l} k \ j\end{array}\right)$ 等等
$$
\开始{对齐}
&=|h| \sum_{k=2}^{\infty}\left|a_{k}\right|\left(\sum_{j=0}^{k-2}\left(\begin{array}{c}
k \
j
\end{数组}\right)|x-a|^{j}|h|^{(k-2)-j}\right) \
&\leq|h| \sum_{k=2}^{\infty}\left|a_{k}\right| k(k-1) \sum_{j=0}^{k-2}\left(\begin{数组}{c}
k-2 \
j
\end{数组}\right)|x-a|^{j}|h|^{(k-2)-j}
\end{对齐}
$$
$=|h| \sum_{k=2}^{\infty}\left|a_{k}\right| k(k-1)(|x-a|+|h|)^{k-2}<|h| \sum_{k=2}^{\infty}\left|a_{k}\right| k(k-1)$ 假设和刚刚观察到的关于 $\lim {k \rightarrow \infty} k^{1 / k}$ 通过假设和刚刚观察到的关于 $\lim {k \rightarrow \infty} k^{1 / k}$, $$ \lim \sup {k \rightarrow \infty}\left(\left|a{ k}\right| k(k-1) r^{k-2}\right)^{1 / k}<1 $$
$$
\left|\frac{f(x+h)-f(x)}{h}-\sum_{k=1}^{\infty} a_{k} k(xa)^{k-1}\right |<C|h|
$$

微积分note Integer Multiples of Irrational Numbers 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。