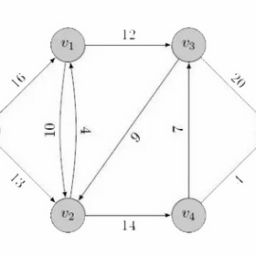
运筹学(Operation)是近代应用数学的一个分支。它把具体的问题进行数学抽象,然后用像是统计学、数学模型和算法等方法加以解决,以此来寻找复杂问题中的最佳或近似最佳的解答。
作为专业的留学生服务机构,Assignmentexpert™多年来已为美国、英国、加拿大、澳洲等留学热门地的学生提供专业的学术服务,包括但不限于论文代写,A作业代写,Dissertation代写,Report代写,Paper代写,Presentation代写,网课代修等等。为涵盖高中,本科,研究生等海外留学生提供辅导服务,辅导学科包括数学,物理,统计,化学,金融,经济学,会计学等全球99%专业科目。写作团队既有专业英语母语作者,也有海外名校硕博留学生,每位写作老师都拥有过硬的语言能力,专业的学科背景和学术写作经验。我们承诺100%原创,100%专业,100%准时,100%满意。
my-assignmentexpert愿做同学们坚强的后盾,助同学们顺利完成学业,同学们如果在学业上遇到任何问题,请联系my-assignmentexpert™,我们随时为您服务!
运筹学代写
We first introduce the notion of “interior” of cones.
Definition 1 We call $\mathbf{X}$ an interior point of cone $K$ if and only if, for any point $\mathbf{Y} \in K^{*}, \mathbf{Y} \bullet \mathbf{X}=0$ implies $\mathbf{Y}=\mathbf{0}$.
The set of interior points of $K$ is denoted by $\stackrel{\circ}{K}$.
Theorem 1 The interior of the following convex cones are given as:
- The interior of the nonnegative orthant cone is the set of all vectors where every entry is
positive. - The interior of the positive semidefinite cone is the set of all positive definite matrices.
- The interior of $p$-order cone is the set of $\left{(u ; x) \in E^{n+1} \quad: u>|\mathbf{x}|{p}\right}$. We give a sketch of the proof for the second-order cone, i.e., $p=2$. Let $(\bar{u} ; \overline{\mathbf{x}}) \neq$ 0 be any second-order cone point but $\bar{u}=|\overrightarrow{\mathbf{x}}|$. Then, we can choose a dual cone (also the second-order cone) point $(v ; y)$ such that $$ v=\alpha \bar{u}, \mathbf{y}=-\alpha \overline{\mathbf{x}} $$ for a positive $\alpha$. Note that $$ (\bar{u} ; \overline{\mathbf{x}}) \bullet(v ; \mathbf{y})=\alpha \bar{v}^{2}-\alpha|\overline{\mathbf{x}}|^{2}=0 . $$ Then, one can let $\alpha>0$ so that $(v ; \mathbf{y})$ cannot be zero Now let $(\bar{u} ; \overline{\mathbf{x}})$ be any given second-order cone point with $\bar{u}>|\overline{\mathbf{x}}|$. We like to prove that, for any dual cone (also the second-order cone) point $(v ; \mathbf{y})$, $(\bar{u} ; \overline{\mathbf{x}})$ $(\bar{u} ; \overline{\mathbf{x}}) \bullet(v ; \mathbf{y})=0$ 173 Proposition 1 Let $\mathbf{X} \in K$ and $\mathbf{Y} \in K^{} .$ Then For any nonnegative constant $\kappa, \mathbf{Y} \cdot \mathbf{X} \leq \kappa$ where the interior of the feasible region is $$ \stackrel{\circ}{\mathcal{F}}:={\mathbf{X}: \mathcal{A} \mathbf{X}=\mathbf{b}, \mathbf{X} \in \stackrel{\circ}{K}} $$ If $\mathcal{F}$ is empty with $K=E{+}^{n}$, from Farkas’ lemma for linear programming, a vector $\mathbf{y} \in E^{m}$, with $\mathbf{y}^{T} \mathbf{A} \leq \mathbf{0}$ and $\mathbf{y}^{T} \mathbf{b}>0$, always exists and is called an infeasibility certificate for the system ${\mathbf{x}: \mathbf{A x}=\mathbf{b}, \mathbf{x} \geq 0}$.
Does this alternative relations hold for $\bar{K}$ being a general closed convex one? Let us rigorousize the question. Let us define the reverse operator of (6.2) from a vector to a matrix:
(6.4)
$\mathbf{y}^{T} \mathcal{A}=\sum_{i=1}^{m} \mathbf{A}{i} y{i} .$ $\mathbf{X} \in E^{k \times n}$
$$
\mathbf{y}^{T} \mathcal{A} \bullet \mathbf{X}=\mathbf{y}^{T}(\mathcal{A X}),
$$
Note that, by the definition, for any matrix
that is, the association property holds. Also, $\left(\mathbf{y}^{T} \mathcal{A}\right)^{T}=\mathcal{A}^{T} \mathbf{y}$, that is, the transpose operation applies here as well.
Then, the question becomes: when $\mathcal{F}$ is empty, does there exist a vector $\mathbf{y} \in$ $E^{m}$ such that $-\mathbf{y}^{T} \mathcal{A} \in K^{}$ and $\mathbf{y}^{T} \mathbf{b}>0$ ? Similarly, one can ask: when set $\left{\mathbf{y}: \mathbf{C}^{T}-\mathbf{y}^{T} \mathcal{A} \in K\right}$ is empty, does there exist a matrix $\mathbf{X} \in K^{*}$ such that $\mathcal{A} \mathbf{X}=\mathbf{0}$ and $\mathbf{C} \cdot \mathbf{X}<0$ ? Note that the answer to the second question is also “yes” when $K=E_{+}^{n}$. $K=E_{+}^{n}$
Example below.

- 非负正锥的内部是所有向量的集合,其中每个条目都是正的。
- 正半定锥的内部是所有正定矩阵的集合.
- $p$-order 锥的内部是 $\left{(u ; x) \in E^{n+1} \quad: u>|\mathbf{x} |{p}\right}$。我们给出了二阶锥的证明草图,即 $p=2$。令 $(\bar{u} ; \overline{\mathbf{x}}) \neq$ 0 为任何二阶锥点,但 $\bar{u}=|\overrightarrow{\mathbf{x}}|$ .然后,我们可以选择一个双锥(也是二阶锥)点 $(v ; y)$ 使得 $$ v=\alpha \bar{u}, \mathbf{y}=-\alpha \overline{ \mathbf{x}} $$ 为正的 $\alpha$。注意 $$ (\bar{u} ; \overline{\mathbf{x}}) \bullet(v ; \mathbf{y})=\alpha \bar{v}^{2}-\alpha|\overline {\mathbf{x}}|^{2}=0 。 $$ 那么,可以让 $\alpha>0$ 使得 $(v ; \mathbf{y})$ 不能为零 现在让 $(\bar{u} ; \overline{\mathbf{x}})$是具有 $\bar{u}>|\overline{\mathbf{x}}|$ 的任何给定二阶锥点。我们想证明,对于任何双锥(也是二阶锥)点 $(v ; \mathbf{y})$, $(\bar{u} ; \overline{\mathbf{x}})$ $(\bar{u} ; \overline{\mathbf{x}}) \bullet(v ; \mathbf{y})=0$ 173 命题 1 令 $\mathbf{X} \in K$ 和 $\mathbf {Y} \in K^{} .$ 那么对于任何非负常数 $\kappa,\mathbf{Y} \cdot \mathbf{X} \leq \kappa$ 其中可行域的内部是 $$ \stackrel{ \circ}{\mathcal{F}}:={\mathbf{X}: \mathcal{A} \mathbf{X}=\mathbf{b}, \mathbf{X} \in \stackrel{\circ}{ K}} $$ 如果 $\mathcal{F}$ 为空且 $K=E{+}^{n}$,来自 Farkas 的线性规划引理,向量 $\mathbf{y} \in E^{ m}$,$\mathbf{y}^{T} \mathbf{A} \leq \mathbf{0}$ 和 $\mathbf{y}^{T} \mathbf{b}>0$,总是存在并被称为系统 ${\mathbf{x} 的不可行证书: \mathbf{A x}=\mathbf{b}, \mathbf{x} \geq 0}$。
这种替代关系是否成立$\bar{K}$ 是一个一般闭凸的?让我们把问题严格化。让我们定义从向量到矩阵的 (6.2) 的逆算子:
(6.4)
$\mathbf{y}^{T} \mathcal{A}=\sum_{i=1}^ {m} \mathbf{A}{i} y{i} .$ $\mathbf{X} \in E^{k \times n}$
$$
\mathbf{y}^{T } \mathcal{A} \bullet \mathbf{X}=\mathbf{y}^{T}(\mathcal{AX}),
$$
注意,根据定义,对于任何矩阵< br>也就是说,关联属性成立。还有$\left(\mathbf{y}^{T} \mathcal{A}\right)^{T}=\mathcal{A}^{T} \mathbf{y}$,也就是转置操作这里也适用。
那么问题就变成了:当 $\mathcal{F}$ 为空时,是否存在一个向量 $\mathbf{y} \in$ $E^{m}$ 使得 $- \mathbf{y}^{T} \mathcal{A} \in K^{}$ 和 $\mathbf{y}^{T} \mathbf{b}>0$ ?类似地,人们可以问:当设置 $\left{\mathbf{y}: \mathbf{C}^{T}-\mathbf{y}^{T} \mathcal{A} \in K\right}$ 是空,是否存在矩阵 $\mathbf{X} \in K^{*}$ 使得 $\mathcal{A} \mathbf{X}=\mathbf{0}$ 和 $\mathbf{C} \cdot \mathbf{X}<0$ ?请注意,当 $K=E_{+}^{n}$ 时,第二个问题的答案也是“是”。 $K=E_{+}^{n}$
示例如下。
运筹学代考

什么是运筹学代写
运筹学(OR)是一种解决问题和决策的分析方法,在组织管理中很有用。在运筹学中,问题被分解为基本组成部分,然后通过数学分析按定义的步骤解决。
运筹学的过程大致可以分为以下几个步骤:
- 确定需要解决的问题。
- 围绕问题构建一个类似于现实世界和变量的模型。
- 使用模型得出问题的解决方案。
- 在模型上测试每个解决方案并分析其成功。
- 实施解决实际问题的方法。
与运筹学交叉的学科包括统计分析、管理科学、博弈论、优化理论、人工智能和复杂网络分析。所有这些学科的目标都是解决某一个现实中出现的复杂问题或者用数学的方法为决策提供指导。 运筹学的概念是在二战期间由参与战争的数学家们提出的。二战后,他们意识到在运筹学中使用的技术也可以被应用于解决商业、政府和社会中的问题。
运筹学代写的三个特点
所有运筹学解决实际问题的过程中都具有三个主要特征:
- 优化——运筹学的目的是在给定的条件下达到某一机器或者模型的最佳性能。优化还涉及比较不同选项和缩小潜在最佳选项的范围。
- 模拟—— 这涉及构建模型,以便在应用解决方案刀具体的复杂大规模问题之前之前尝试和测试简单模型的解决方案。
- 概率和统计——这包括使用数学算法和数据挖掘来发现有用的信息和潜在的风险,做出有效的预测并测试可能的解决方法。
运筹学领域提供了比普通软件和数据分析工具更强大的决策方法。此外,运筹学可以根据特定的业务流程或用例进行定制,以确定哪些技术最适合解决问题。
运筹学可以应用于各种活动,比如:计划和时间管理(Planning and Time Management),城乡规划(Urban and Rural Planning),企业资源计划(ERP)与供应链管理(Supply Chain Management)等等。 如有代写代考需求,欢迎同学们联系Assignmentexpert™,我们期待为你服务!