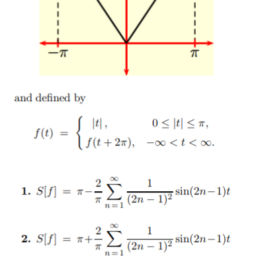
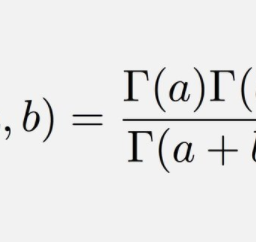10.3 The Gamma Function
Recall the definition of an improper integral specialized to $[0, \infty) . \int_{0}^{\infty} f(t) d t$ is the number, if it exists which equals $\lim {\delta \rightarrow 0, R \rightarrow \infty} \int{\delta}^{R} f(t) d t$. Equivalently, if $\delta_{n} \rightarrow 0$ and $R_{n} \rightarrow \infty$ are any two sequences converging to 0 then $\int_{\delta_{n}}^{R_{n}} f(t) d t \rightarrow \int_{0}^{\infty} f(t) d t$
Definition 10.3.1 The gamma function is defined by $\Gamma(\alpha) \equiv \int_{0}^{\infty} e^{-t} t^{\alpha-1} d t$ whenever $\alpha>0$.
Lemma 10.3.2 The improper integral $\int_{0}^{\infty} e^{-t} t^{\alpha-1} d t$ exists for each $\alpha>0$.
Proof: Say $\sigma, \delta$ are small and positive and $R, M$ are large. Then say $\sigma<\delta$ and $R<M$. Then
$$
\begin{aligned}
\left|\int_{\delta}^{R} e^{-t} t^{\alpha-1} d t-\int_{\sigma}^{M} e^{-t} t^{\alpha-1}\right| & \leq \int_{R}^{M} e^{-t} t^{\alpha-1} d t+\int_{\sigma}^{\delta} e^{-t} t^{\alpha-1} d t \
& \leq e^{-R}+\frac{1}{\alpha}\left(\delta^{\alpha}-\sigma^{\alpha}\right)
\end{aligned}
$$
which is small if $R$ is large and $\delta$ is small. Similar reasoning shows that
$$
\left|\int_{\sigma}^{R} e^{-t} t^{\alpha-1} d t-\int_{\delta}^{M} e^{-t} t^{\alpha-1}\right|,\left|\int_{\delta}^{M} e^{-t} t^{\alpha-1} d t-\int_{\sigma}^{R} e^{-t} t^{\alpha-1}\right|
$$
is small. Thus if $\delta_{n} \rightarrow 0+$ and $R_{n} \rightarrow \infty,\left{\int_{\delta_{n}}^{R_{n}} e^{-t} t^{\alpha-1} d t\right}$ is a Cauchy sequence and that which it converges to is not dependent on the choice of sequence $\delta_{n} \rightarrow 0$ and $R_{n} \rightarrow \infty$.
This gamma function has some fundamental properties described in the following proposition. In case the improper integral exists, we can obviously compute it in the form $\lim {\delta \rightarrow 0+} \int{\delta}^{1 / \delta} f(t) d t$ which is used in what follows. Thus also the usual algebraic properties of the Riemann integral are inherited by the improper integral.
Proposition 10.3.3 For $n$ a positive integer, $n !=\Gamma(n+1) .$ In general, $\Gamma(1)=$ $1, \Gamma(\alpha+1)=\alpha \Gamma(\alpha)$
Proof: First of all, $\Gamma(1)=\lim {\delta \rightarrow 0} \int{\delta}^{\delta^{-1}} e^{-t} d t=\lim {\delta \rightarrow 0}\left(e^{-\delta}-e^{-\left(\delta^{-1}\right)}\right)=1 .$ Next, for $\alpha>0$, $$ \begin{gathered} \Gamma(\alpha+1)=\lim {\delta \rightarrow 0} \int_{\delta}^{\delta^{-1}} e^{-t} t^{\alpha} d t=\lim {\delta \rightarrow 0}\left[-\left.e^{-t} t^{\alpha}\right|{\delta} ^{\delta^{-1}}+\alpha \int_{\delta}^{\delta^{-1}} e^{-t} t^{\alpha-1} d t\right] \
=\lim {\delta \rightarrow 0}\left(e^{-\delta} \delta^{\alpha}-e^{-\left(\delta^{-1}\right)} \delta^{-\alpha}+\alpha \int{\delta}^{\delta^{-1}} e^{-t} t^{\alpha-1} d t\right)=\alpha \Gamma(\alpha)
\end{gathered}
$$
Now it is defined that $0 !=1$ and so $\Gamma(1)=0 !$. Suppose that $\Gamma(n+1)=n !$, what of $\Gamma(n+2) ?$ Is it $(n+1) ! ?$ if so, then by induction, the proposition is established. From what was just shown, $\Gamma(n+2)=\Gamma(n+1)(n+1)=n !(n+1)=(n+1) !$ and so this proves the proposition.
The properties of the gamma function also allow for a fairly easy proof about differentiating under the integral in a Laplace transform. First is a definition.
Definition 10.3.4 A function $\phi$ has exponential growth on $[0, \infty)$ if there are positive constants $\lambda, C$ such that $|\phi(t)| \leq C e^{\lambda t}$ for all $t \geq 0$.
10.3 The Gamma Function
Recall the definition of an improper integral specialized to $[0, \infty) . \int_{0}^{\infty} f(t) d t$ is the number, if it exists which equals $\lim {\delta \rightarrow 0, R \rightarrow \infty} \int{\delta}^{R} f(t) d t$. Equivalently, if $\delta_{n} \rightarrow 0$ and $R_{n} \rightarrow \infty$ are any two sequences converging to 0 then $\int_{\delta_{n}}^{R_{n}} f(t) d t \rightarrow \int_{0}^{\infty} f(t) d t$
Definition 10.3.1 The gamma function is defined by $\Gamma(\alpha) \equiv \int_{0}^{\infty} e^{-t} t^{\alpha-1} d t$ whenever $\alpha>0$.
Lemma 10.3.2 The improper integral $\int_{0}^{\infty} e^{-t} t^{\alpha-1} d t$ exists for each $\alpha>0$.
Proof: Say $\sigma, \delta$ are small and positive and $R, M$ are large. Then say $\sigma<\delta$ and $R<M$. Then
$$
\begin{aligned}
\left|\int_{\delta}^{R} e^{-t} t^{\alpha-1} d t-\int_{\sigma}^{M} e^{-t} t^{\alpha-1}\right| & \leq \int_{R}^{M} e^{-t} t^{\alpha-1} d t+\int_{\sigma}^{\delta} e^{-t} t^{\alpha-1} d t \
& \leq e^{-R}+\frac{1}{\alpha}\left(\delta^{\alpha}-\sigma^{\alpha}\right)
\end{aligned}
$$
which is small if $R$ is large and $\delta$ is small. Similar reasoning shows that
$$
\left|\int_{\sigma}^{R} e^{-t} t^{\alpha-1} d t-\int_{\delta}^{M} e^{-t} t^{\alpha-1}\right|,\left|\int_{\delta}^{M} e^{-t} t^{\alpha-1} d t-\int_{\sigma}^{R} e^{-t} t^{\alpha-1}\right|
$$
is small. Thus if $\delta_{n} \rightarrow 0+$ and $R_{n} \rightarrow \infty,\left{\int_{\delta_{n}}^{R_{n}} e^{-t} t^{\alpha-1} d t\right}$ is a Cauchy sequence and that which it converges to is not dependent on the choice of sequence $\delta_{n} \rightarrow 0$ and $R_{n} \rightarrow \infty$.
This gamma function has some fundamental properties described in the following proposition. In case the improper integral exists, we can obviously compute it in the form $\lim {\delta \rightarrow 0+} \int{\delta}^{1 / \delta} f(t) d t$ which is used in what follows. Thus also the usual algebraic properties of the Riemann integral are inherited by the improper integral.
Proposition 10.3.3 For $n$ a positive integer, $n !=\Gamma(n+1) .$ In general, $\Gamma(1)=$ $1, \Gamma(\alpha+1)=\alpha \Gamma(\alpha)$
Proof: First of all, $\Gamma(1)=\lim {\delta \rightarrow 0} \int{\delta}^{\delta^{-1}} e^{-t} d t=\lim {\delta \rightarrow 0}\left(e^{-\delta}-e^{-\left(\delta^{-1}\right)}\right)=1 .$ Next, for $\alpha>0$, $$ \begin{gathered} \Gamma(\alpha+1)=\lim {\delta \rightarrow 0} \int_{\delta}^{\delta^{-1}} e^{-t} t^{\alpha} d t=\lim {\delta \rightarrow 0}\left[-\left.e^{-t} t^{\alpha}\right|{\delta} ^{\delta^{-1}}+\alpha \int_{\delta}^{\delta^{-1}} e^{-t} t^{\alpha-1} d t\right] \
=\lim {\delta \rightarrow 0}\left(e^{-\delta} \delta^{\alpha}-e^{-\left(\delta^{-1}\right)} \delta^{-\alpha}+\alpha \int{\delta}^{\delta^{-1}} e^{-t} t^{\alpha-1} d t\right)=\alpha \Gamma(\alpha)
\end{gathered}
$$
Now it is defined that $0 !=1$ and so $\Gamma(1)=0 !$. Suppose that $\Gamma(n+1)=n !$, what of $\Gamma(n+2) ?$ Is it $(n+1) ! ?$ if so, then by induction, the proposition is established. From what was just shown, $\Gamma(n+2)=\Gamma(n+1)(n+1)=n !(n+1)=(n+1) !$ and so this proves the proposition.
The properties of the gamma function also allow for a fairly easy proof about differentiating under the integral in a Laplace transform. First is a definition.
Definition 10.3.4 A function $\phi$ has exponential growth on $[0, \infty)$ if there are positive constants $\lambda, C$ such that $|\phi(t)| \leq C e^{\lambda t}$ for all $t \geq 0$.
回想一下专用于 $[0, \infty) 的不正确积分的定义。 \int_{0}^{\infty} f(t) dt$ 是数字,如果它存在等于 $\lim {\delta \rightarrow 0, R \rightarrow \infty} \int{\delta}^{ R} f(t) dt$。等效地,如果 $\delta_{n} \rightarrow 0$ 和 $R_{n} \rightarrow \infty$ 是收敛到 0 的任意两个序列,则 $\int_{\delta_{n}}^{R_{n}} f (t) dt \rightarrow \int_{0}^{\infty} f(t) dt$
定义 10.3.1 伽马函数由 $\Gamma(\alpha) \equiv \int_{0}^{\infty} e^{-t} t^{\alpha-1} dt$ 定义,只要 $\alpha> 0 美元。
引理 10.3.2 对于每个 $\alpha>0$,存在不正确的积分 $\int_{0}^{\infty} e^{-t} t^{\alpha-1} d t$。
证明:假设$\sigma, \delta$ 是小而正的,$R, M$ 是大的。然后说 $\sigma<\delta$ 和 $R<M$。然后
$$
\开始{对齐}
\left|\int_{\delta}^{R} e^{-t} t^{\alpha-1} d t-\int_{\sigma}^{M} e^{-t} t^{\ alpha-1}\对| & \leq \int_{R}^{M} e^{-t} t^{\alpha-1} d t+\int_{\sigma}^{\delta} e^{-t} t^{\alpha -1} dt \
& \leq e^{-R}+\frac{1}{\alpha}\left(\delta^{\alpha}-\sigma^{\alpha}\right)
\end{对齐}
$$
如果 $R$ 很大并且 $\delta$ 很小,则它很小。类似的推理表明
$$
\left|\int_{\sigma}^{R} e^{-t} t^{\alpha-1} d t-\int_{\delta}^{M} e^{-t} t^{\ alpha-1}\right|,\left|\int_{\delta}^{M} e^{-t} t^{\alpha-1} d t-\int_{\sigma}^{R} e^ {-t} t^{\alpha-1}\right|
$$
是小。因此如果 $\delta_{n} \rightarrow 0+$ 和 $R_{n} \rightarrow \infty,\left{\int_{\delta_{n}}^{R_{n}} e^{-t} t^{\alpha-1} dt\right}$ 是一个柯西序列,它收敛到的序列不依赖于序列 $\delta_{n} \rightarrow 0$ 和 $R_{n} \rightarrow 的选择\infty$。
该伽马函数具有以下命题中描述的一些基本属性。如果存在不正确的积分,我们显然可以以 $\lim {\delta \rightarrow 0+} \int{\delta}^{1 / \delta} f(t) dt$ 的形式计算它接下来是什么。因此,黎曼积分的通常代数性质也被不正确积分所继承。
命题 10.3.3 对于 $n$ 一个正整数,$n !=\Gamma(n+1) .$ 一般来说,$\Gamma(1)=$ $1, \Gamma(\alpha+1)=\alpha \伽玛(\alpha)$
证明:首先,$\Gamma(1)=\lim {\delta \rightarrow 0} \int{\delta}^{\delta^{-1}} e^{-t} dt=\lim _ {\delta \rightarrow 0}\left(e^{-\delta}-e^{-\left(\delta^{-1}\right)}\right)=1 .$ 接下来,对于 $\alpha> 0$,
$$
\开始{聚集}
\Gamma(\alpha+1)=\lim {\delta \rightarrow 0} \int{\delta}^{\delta^{-1}} e^{-t} t^{\alpha} dt=\ lim {\delta \rightarrow 0}\left[-\left.e^{-t} t^{\alpha}\right|{\delta} ^{\delta^{-1}}+\alpha \ int_{\delta}^{\delta^{-1}} e^{-t} t^{\alpha-1} dt\right] \
=\lim {\delta \rightarrow 0}\left(e^{-\delta} \delta^{\alpha}-e^{-\left(\delta^{-1}\right)} \delta^ {-\alpha}+\alpha \int{\delta}^{\delta^{-1}} e^{-t} t^{\alpha-1} dt\right)=\alpha \Gamma(\alpha )
\结束{聚集}
$$
现在定义 $0 !=1$ 等 $\Gamma(1)=0 !$。假设 $\Gamma(n+1)=n !$,那么 $\Gamma(n+2) 呢?$ 是 $(n+1) 吗! ?$ 如果是,那么通过归纳,命题成立。从刚刚展示的内容来看,$\Gamma(n+2)=\Gamma(n+1)(n+1)=n !(n+1)=(n+1) !$ 所以这证明了这个命题。
伽马函数的属性也允许在拉普拉斯变换的积分下进行微分的相当简单的证明。首先是一个定义。
定义 10.3.4 如果存在正常数 $\lambda, C$ 使得 $|\phi(t)| 函数 $\phi$ 在 $[0, \infty)$ 上呈指数增长\leq C e^{\lambda t}$ 对于所有 $t \geq 0$。
10.3 伽玛函数
回想一下专用于 $[0, \infty) 的不正确积分的定义。 \int_{0}^{\infty} f(t) dt$ 是数字,如果它存在等于 $\lim {\delta \rightarrow 0, R \rightarrow \infty} \int{\delta}^{ R} f(t) dt$。等效地,如果 $\delta_{n} \rightarrow 0$ 和 $R_{n} \rightarrow \infty$ 是收敛到 0 的任意两个序列,则 $\int_{\delta_{n}}^{R_{n}} f (t) dt \rightarrow \int_{0}^{\infty} f(t) dt$
定义 10.3.1 伽马函数由 $\Gamma(\alpha) \equiv \int_{0}^{\infty} e^{-t} t^{\alpha-1} dt$ 定义,只要 $\alpha> 0 美元。
引理 10.3.2 对于每个 $\alpha>0$,存在不正确的积分 $\int_{0}^{\infty} e^{-t} t^{\alpha-1} d t$。
证明:假设$\sigma, \delta$ 是小而正的,$R, M$ 是大的。然后说 $\sigma<\delta$ 和 $R<M$。然后
$$
\开始{对齐}
\left|\int_{\delta}^{R} e^{-t} t^{\alpha-1} d t-\int_{\sigma}^{M} e^{-t} t^{\ alpha-1}\对| & \leq \int_{R}^{M} e^{-t} t^{\alpha-1} d t+\int_{\sigma}^{\delta} e^{-t} t^{\alpha -1} dt \
& \leq e^{-R}+\frac{1}{\alpha}\left(\delta^{\alpha}-\sigma^{\alpha}\right)
\end{对齐}
$$
如果 $R$ 很大并且 $\delta$ 很小,则它很小。类似的推理表明
$$
\left|\int_{\sigma}^{R} e^{-t} t^{\alpha-1} d t-\int_{\delta}^{M} e^{-t} t^{\ alpha-1}\right|,\left|\int_{\delta}^{M} e^{-t} t^{\alpha-1} d t-\int_{\sigma}^{R} e^ {-t} t^{\alpha-1}\right|
$$
是小。因此如果 $\delta_{n} \rightarrow 0+$ 和 $R_{n} \rightarrow \infty,\left{\int_{\del

微积分note Integer Multiples of Irrational Numbers 请认准UprivateTA™. UprivateTA™为您的留学生涯保驾护航。