如果你也在 怎样代写运筹学Operations Research这个学科遇到相关的难题,请随时右上角联系我们的24/7代写客服。假设检验Hypothesis是假设检验是统计学中的一种行为,分析者据此检验有关人口参数的假设。分析师采用的方法取决于所用数据的性质和分析的原因。假设检验是通过使用样本数据来评估假设的合理性。
运筹学(Operation)是近代应用数学的一个分支。它把具体的问题进行数学抽象,然后用像是统计学、数学模型和算法等方法加以解决,以此来寻找复杂问题中的最佳或近似最佳的解答。
二战中运筹学的应用
在二战时期,作战研究被定义为 “一种科学方法,为执行部门提供有关其控制的行动的决策的量化依据”。它的其他名称包括作战分析(英国国防部从1962年开始)和定量管理。
在第二次世界大战期间,英国有近1000名男女从事作战研究。大约有200名作战研究科学家为英国军队工作。
帕特里克-布莱克特在战争期间为几个不同的组织工作。战争初期,在为皇家飞机研究所(RAE)工作时,他建立了一个被称为 “马戏团 “的团队,帮助减少了击落一架敌机所需的防空炮弹数量,从不列颠战役开始时的平均超过20,000发减少到1941年的4,000发。
my-assignmentexpert™ 运筹学Operations Research作业代写,免费提交作业要求, 满意后付款,成绩80\%以下全额退款,安全省心无顾虑。专业硕 博写手团队,所有订单可靠准时,保证 100% 原创。my-assignmentexpert™, 最高质量的运筹学Operations Research作业代写,服务覆盖北美、欧洲、澳洲等 国家。 在代写价格方面,考虑到同学们的经济条件,在保障代写质量的前提下,我们为客户提供最合理的价格。 由于统计Statistics作业种类很多,同时其中的大部分作业在字数上都没有具体要求,因此运筹学Operations Research作业代写的价格不固定。通常在经济学专家查看完作业要求之后会给出报价。作业难度和截止日期对价格也有很大的影响。
想知道您作业确定的价格吗? 免费下单以相关学科的专家能了解具体的要求之后在1-3个小时就提出价格。专家的 报价比上列的价格能便宜好几倍。
my-assignmentexpert™ 为您的留学生涯保驾护航 在运筹学Operations Research作业代写方面已经树立了自己的口碑, 保证靠谱, 高质且原创的应用数学applied math代写服务。我们的专家在运筹学Operations Research代写方面经验极为丰富,各种运筹学Operations Research相关的作业也就用不着 说。
我们提供的假设检验Hypothesis及其相关学科的代写,服务范围广, 其中包括但不限于:
- 商业分析 Business Analysis
- 计算机科学 Computer Science
- 数据挖掘/数据科学/大数据 Data Mining / Data Science / Big Data
- 决策分析 Decision Analytics
- 金融工程 Financial Engineering
- 数据预测 Data Forecasting
- 博弈论 Game Theory
- 地理/地理信息科学 Geography/Geographic Information Science
- 图论 Graph Theory
- 工业工程 Industrial Engineering
- 库存控制 Inventory control
- 数学建模 Mathematical Modeling
- 数学优化 Mathematical Optimization
- 概率和统计 Probability and statistics
- 排队论 Queueing theory
- 社交网络/交通预测模型 Social network/traffic prediction modeling
- 随机过程 Stochastic processes
- 供应链管理 Supply chain management
运筹学代写
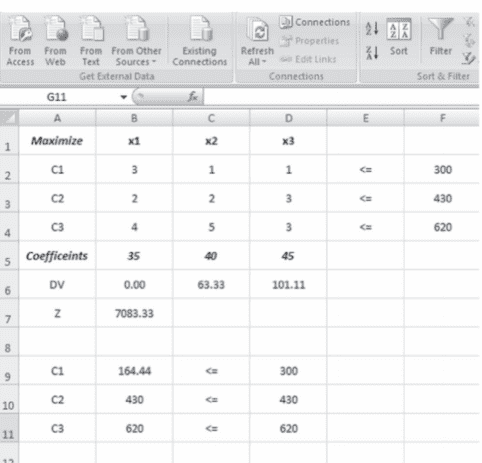
数学代写|运筹学作业代写OPERATIONS RESEARCH代考|Illustration of Sensitivity Analysis
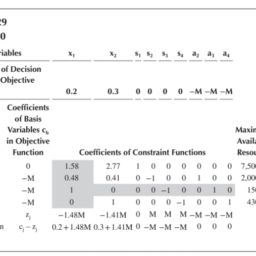
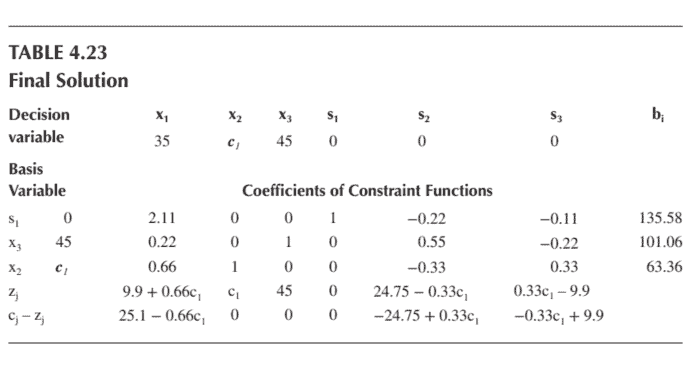
i. Range of feasibility for $\mathbf{b}{1}$ : Feasibility condition states that values in RHS column should be greater or equal to zero. So, to estimate the allowable range of $b{1}$, which indicates the maximum resource available and is associated with slack variable $s_{1}$, the following formula is used.
$\mathrm{b}{1}$ value in final solution $+$ (change in $\mathrm{b}{1}, \Delta \mathrm{b}_{1}$ )
- (corresponding value of slack variable) $\geq 0$
Coefficients in the slack variable column indicate change in corresponding basic variable with the introduction of that slack variable in the solution. For instance, in case of $\mathrm{s}{1}$, if one unit of it is introduced in the solution, then corresponding change in $b{1}$ would be to the amount of coefficients of basic variables indicated in $\mathrm{s}{1}$ column. So, if coefficient in $\mathrm{s}{1}$ column is zero, then it would imply that $b_{1}$ would change by zero as corresponding basic variable has changed by zero. Hence, in this case $b_{1}$ is insensitive parameter and its value is equivalent to slack variable $\mathrm{s}{1}$. Any change in its value would keep $\mathrm{s}{1}$ same as values of corresponding basic variables in associated $s_{1}$ column are zero. For instance, in constraint equation of $\mathrm{b}{1}$, the value of $\mathrm{s}{1}$ is deduced to be $135.58$. Now if it is increased by one unit, then corresponding values of basic variables in the $s_{1}$ column, which are $s_{1}, x_{2}$ and $x_{3}$, imply a change in values of $x_{2}$ and $x_{3}$ as zero, and the changed value of $b_{1}$ would be equivalent to change in $\mathrm{s}{1}$, i.e. one unit. So, $b{1}$ becomes insensitive. This is shown below:
$$
\mathrm{Cl}: 3 \mathrm{x}{1}+1 \mathrm{x}{2}+1 \mathrm{x}{3}+1 \mathrm{~s}{1}=300
$$
$$
3 * 0+1 * 63.36+1 * 101.06+1 \mathrm{~s}{1}=164.42 $$ So $\mathrm{s}{1}=135.58$
Thus, putting $\mathrm{x}{1}=0, \mathrm{x}{2}=63.36$ and $\mathrm{x}{3}=101.06$ value of $\mathrm{s}{1}$ would be 135.58. Now according to coefficients in the $\mathrm{s}{1}$ column, $\mathrm{x}{1}$ has not entered the solution, so it is assumed to be zero; $\mathrm{x}{2}$ and $\mathrm{x}{3}$ have changed by zero so their values remain the same, which would result in the same value of $\mathrm{s}{1}$.This value according to the final solution table represents $b{1}$ value. Therefore, $b 1$ becomes an insensitive parameter as any amount introduction of $s_{1}$ would keep $b_{1}$ the same.
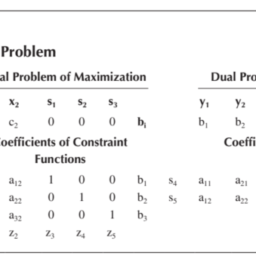
数学代写|运筹学作业代写OPERATIONS RESEARCH代考|illustRation of duality
Maximize $Z=2 x_{1}+6 x_{2}+5 x_{3}$
Subject to:
$$
\begin{gathered}
\mathrm{x}{1}+2 \mathrm{x}{2}+2 \mathrm{x}{3} \leq 10 \ 3 \mathrm{x}{1}+3 \mathrm{x}{2}+2 \mathrm{x}{3} \leq 12 \text { with } \mathrm{x}{1}, \mathrm{x}{2} \geq 0
\end{gathered}
$$
i. Construct the dual form of given primal problem.
ii. Solve only primal by simplex method and identify basic solution at each iteration. Also, identify basic and non-basic variables of dual problem.
Solution:
i. Dual form would be:
Minimize $Z^{*}=10 y_{1}+12 y_{2}$
Subject to:
$$
\begin{gathered}
1 \mathrm{y}{1}+3 \mathrm{y}{2} \geq 2 \
2 \mathrm{y}{1}+3 \mathrm{y}{2} \geq 6 \
2 \mathrm{y}{1}+2 \mathrm{y}{2} \geq 5 \text { with } \mathrm{y}{1}, \mathrm{y}{2} \geq 0
\end{gathered}
$$
ii. Following would be initial models of both primal and dual:
Maximize $\mathrm{Z}=2 \mathrm{x}{1}+6 \mathrm{x}{2}+5 \mathrm{x}{3}+0 \mathrm{~s}{1}+0 \mathrm{~s}{2}$ Subject to: $$ \begin{gathered} x{1}+2 x_{2}+2 x_{3}+1 s_{1}+0 s_{2}=10 \
3 x_{1}+3 x_{2}+2 x_{3}+0 s_{1}+1 s_{2}=12 \text { with } x_{1}, x_{2} \geq 0
\end{gathered}
运筹学代考
数学代写|运筹学作业代写OPERATIONS RESEARCH代考|ILLUSTRATION OF SENSITIVITY ANALYSIS
b v1 1 alue in final solution + (change in b ,∆b )1
- c( ) orresponding value of slack variable ≥ 0
- C○rr和sp○nd一世nGv一种一世你和○Fs一世一种C到v一种r一世一种b一世和 ≥0
松弛变量列中的系数表示在解中引入该松弛变量时相应基本变量的变化。例如,如果Coefficients in the slack variable column indicate change in corresponding basic variable with the introduction of that slack variable in the solution. For instance, in case of $s_{1}$, if one unit of it is introduced in the solution, then corresponding change in $b_{1}$ would be to the amount of coefficients of basic variables indicated in $\mathrm{s}{1}$ column. So, if coefficient in $\mathrm{s}{1}$ column is zero, then it would imply that $b_{1}$ would change by zero as corresponding basic variable has changed by zero. Hence, in this case $\mathrm{b}{1}$ is insensitive parameter and its value is equivalent to slack variable $\mathrm{s}{1}$. Any change in its value would keep $\mathrm{s}{1}$ same as values of corresponding basic variables in associated $s{1}$ column are zero. For instance, in constraint equation of $\mathrm{b}{1}$, the value of $\mathrm{s}{1}$ is deduced to be $135.58$. Now if it is increased by one unit, then corresponding values of basic variables in the $s_{1}$ column, which are $s_{1}, x_{2}$ and $x_{3}$, imply a change in values of $x_{2}$ and $x_{3}$ as zero, and the changed value of $b_{1}$ would be equivalent to change in $s_{1}$, i.e. one unit. So, $b_{1}$ becomes insensitive. This is shown below:
\mathrm{Cl}: 3 \mathrm{x} {1}+1 \mathrm{x} {2}+1 \mathrm{x} {3}+1 \mathrm{~s} {1}=300 - 3 * 0+1 * 63.36+1 * 101.06+1 \mathrm{~s} {1}=164.42 $$ 所以 $\mathrm{s} {1}=135.58
C1: 3x +1 2 1x + 1x +3 1 1s = 300
3* 0 1 + + * 63.36 1*101.06 + = 1s1 164.42
数学代写|运筹学作业代写OPERATIONS RESEARCH代考|ILLUSTRATION OF DUALITY
最大化和=2X1+6X2+5X3
服从:
$$
\begin{gathered}
\mathrm{x} {1}+2 \mathrm{x} {2}+2 \mathrm{x} {3} \leq 10 \ 3 \mathrm{x} {1 }+3 \mathrm{x} {2}+2 \mathrm{x} {3} \leq 12 \text { with } \mathrm{x} {1}, \mathrm{x} {2} \geq 0
Maximize Z = 2×1 2 + 6x + 5×3
Subject to:
x1 2 + 2x + 2×3 ≤ 10
3×1 2 + 3x + 2×3 1 ≤ ≥ 12 with x , x 0
Minimize Z * = 10y +1 2 12y
ii. 以下是原始模型和对偶模型的初始模型:
最大化
y1 2 + 3y 2 ≥
2y1 2 + 3y 6 ≥
2y1 2 + 2y 5 ≥ ≥ with y , 1 2 y 0